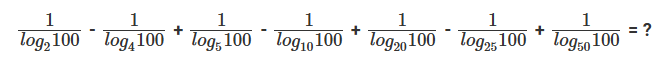Live Updates
• CATKing has launched new chat bot.
• New video on Logs has been released.
495
Learners
asked the doubt
Previous Year Questions
Points A, P, Q and B lie on the same line such that P, Q and B are, respectively, 100 km, 200 km and 300 km away from A. Cars 1 and 2 leave A at the same time and move towards B. Simultaneously, car 3 leaves B and moves towards A. Car 3 meets Car 1 at Q, and Car 2 at P. If each car is moving in uniform speed then the ratio of the speed of Car 2 to that of Car 1 is
Video Explanation
Explanatory Answer
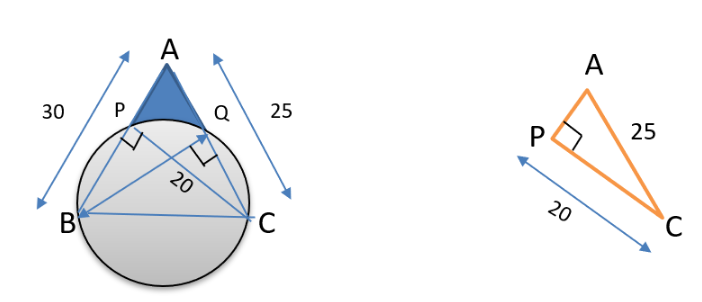
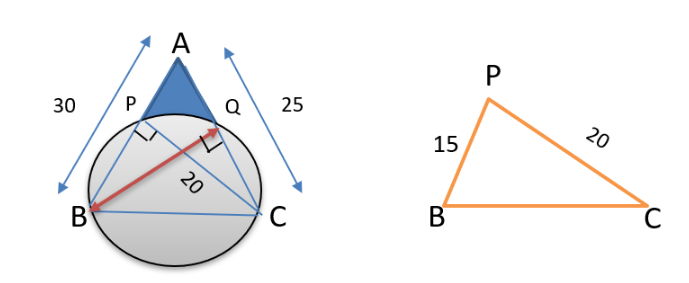
It is given that points A, P, Q and B lie on the same line such that P, Q and B are 100 km, 200 km and 300 km away from A. Let us draw the diagram first. All of them are on the same straight line and P, Q lie between A and B.
Cars 1 and Cars 2 leave A at the same time and move towards B. Simultaneously, Car 3 leaves B and moves towards A. Let us add more details to the diagram. C1 and C2 move towards B and C3 moves towards A. C3 meets C1 at Q and C3 meets C2 at P.
Let us assume the speeds of Car 1, 2, and 3 to be C1, C2 and C3 respectively. Car 1 is travelling quicker than Car 2. When Car 1 travels 200 km to reach Q, Car 3 has travelled 100 km or ratio of their speeds, C1 : C3 is 2 : 1.C1 and C2 move towards B and C3 moves towards A. C3 meets C1 at Q and C3 meets C2 at P.
Let us assume the speeds of Car 1, 2, and 3 to be C1, C2 and C3 respectively.When Car 3 travels 200 km to reach P, Car 2 has travelled 100 km or ratio of their speeds, C3 : C2 is 2 : 1. Now we have all the ratios. We know that C1 : C3 is 2 : 1 and C3 : C2 is 2 : 1. We can see that C3 is the common link
So, taking LCM of C3, we get,
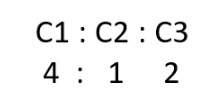
We can see that ratio of speeds of Car 2 to Car 1 is 1 : 4. Hence, C2 : C1 = 1 : 4
Let a1, a2, . , a52 be positive integers such that a1 ＜ a2 ＜ . ＜ a52. Suppose, their arithmetic mean is one less than the arithmetic mean of a2, a3, ., a52. If a52 = 100, then the largest possible value of a1 is
Video Explanation
Explanatory Answer
There are two drums, each containing a mixture of paints A and B. In drum 1, A and B are in the ratio 18 : 7. The mixtures from drums 1 and 2 are mixed in the ratio 3 : 4 and in this final mixture, A and B are in the ratio 13 : 7. In drum 2, then A and B were in the ratio
Video Explanation
Explanatory Answer
Given that two drums each containing a mixture of paints A and B. In drum 1, A and B are in the
ratio 18 : 7. Drums 1 and 2 are mixed in the ratio 3 : 4 and in the final mixture A and B are in the
ratio 13 : 7. For these kinds of questions do not consider separately as A and B. Deal with either A
or B as a share of overall
Here A is 18/25 of overall
In D2, let us assume the proportion of A with respect to the overall mixture is x. 3 parts of D1 is
mixed with parts of D2 to give proportion of A of which is (13/20) th of the overall mixture. From
here on, it is weighted averages.
So, {(18/5)×3 + (x×4)} / 7 = 13/20
54/25 + 4𝑥 = 91/20
4𝑥 = 91/20 - 54/25
4𝑥 = (455−216)/100 = 239/100
𝑥 = 239/400
In drum 2, A is 239/400 so B should be the remaining. Therefore, A : B = 239 : 161
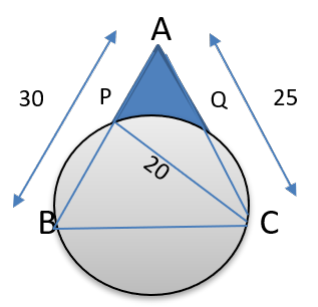
On a triangle ABC, a circle with diameter BC is drawn, intersecting AB and AC at points P and Q, respectively. If the lengths of AB, AC, and CP are 30 cm, 25 cm, and 20 cm respectively, then the length of BQ, in cm, is
Video Explanation
Explanatory Answer
Let t1, t2,… be real numbers such that t1+ t2 +. + tn = 2n2 + 9n + 13, for every positive integer n ≥ 2. If tk=103, then k equals
Video Explanation
Explanatory Answer
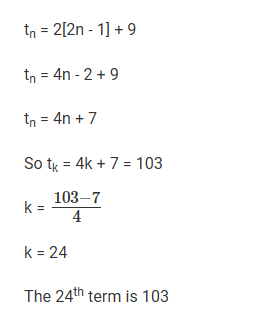
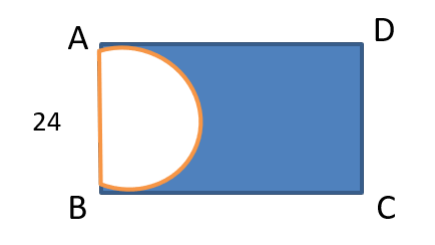
From a rectangle ABCD of area 768 sq cm, a semicircular part with diameter AB and area 72π sq cm is removed. The perimeter of the leftover portion, in cm, is
Video Explanation
Explanatory Answer
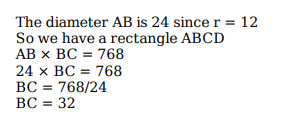
If N and x are positive integers such that NN = 2160 and N2 + 2N is an integral multiple of 2x, then the largest possible x is
Video Explanation
Explanatory Answer
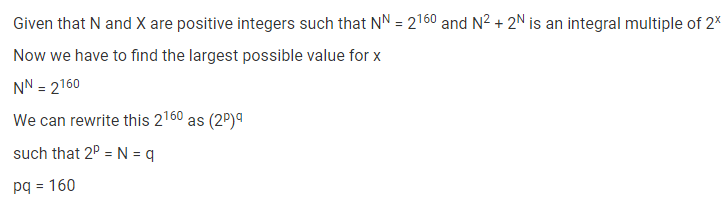
A chord of length 5 cm subtends an angle of 60° at the centre of a circle. The length, in cm, of a chord that subtends an angle of 120° at the centre of the same circle is
Video Explanation
Explanatory Answer
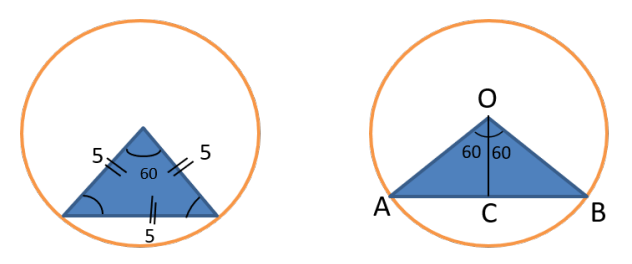
Given that a chord of length 5 cm subtends an angle of 60° at the centre of a circle.
We have to find the length of a chord that subtends an angle of 120° at the centre of the same
circle
sin 60° = BC/OB
⟹ √3/2 = BC/5
⟹ BC = 5√3/2 and AC = 5√3/2
So AB = 5√3/2 + 5√3/2= 5√3
The length of a chord that subtends an angle of 120° at the centre of the same circle is 5√3
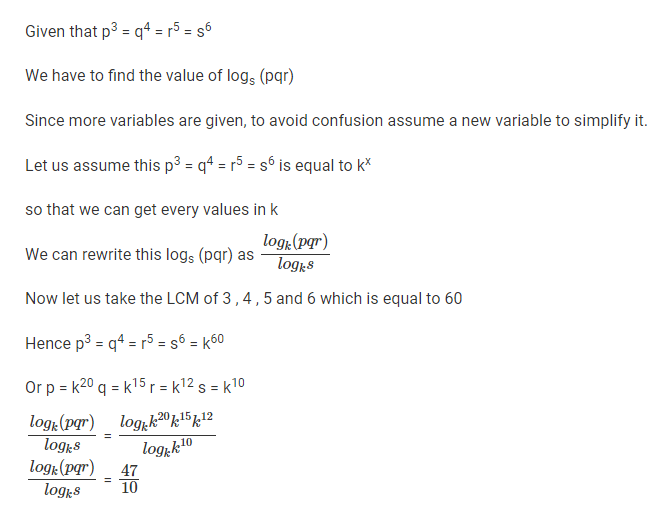
If p3 = q4 = r5 = s6, then the value of logs (pqr) is equal to
Video Explanation
Explanatory Answer
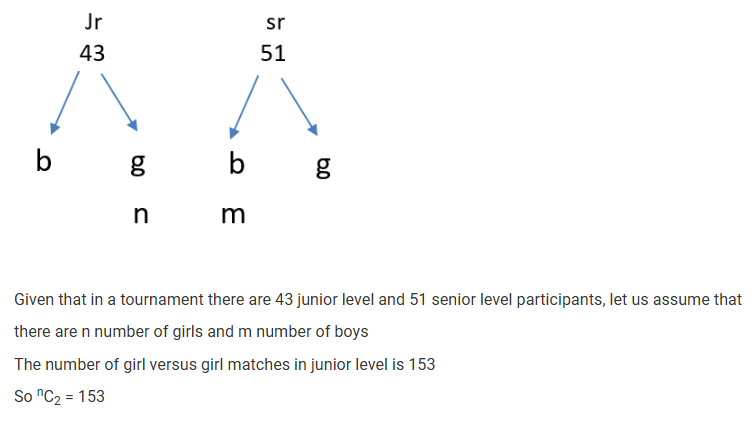
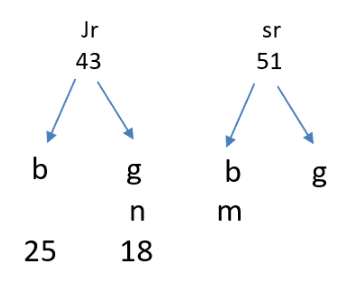
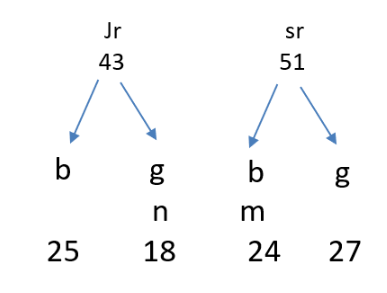
In a tournament, there are 43 junior level and 51 senior level participants. Each pair of juniors play one match. Each pair of seniors play one match. There is no junior versus senior match. The number of girl versus girl matches in junior level is 153, while the number of boy versus boy matches in senior level is 276. The number of matches a boy plays against a girl is
Video Explanation
Explanatory Answer
A 20% ethanol solution is mixed with another ethanol solution, say, S of unknown concentration in the proportion 1:3 by volume. This mixture is then mixed with an equal volume of 20% ethanol solution. If the resultant mixture is a 31.25% ethanol solution, then the unknown concentration of S is
Video Explanation
Explanatory Answer
Given that 20% ethanol solution is mixed with another ethanol solution, say, S of unknown concentration in the proportion 1:3 by volume
This M is then mixed with an equal volume of 20% ethanol solution. If the resultant mixture is a 31.25% ethanol solution ,then the unknown concentration of S has to be found
Equal quantities of mixture and 20% ethanol solution are mixed in equal ratio to get 31.25%
The mixture and 20% ethanol solution are mixed in equal ratio to get 31.25%. This 31.25% is 11.25% more than this 20%. This mixture must be 11.25% more than 31.25% so mixture is equal to 42.5%
This mixture 42.5% is mixed in the ratio 1:3
Using allegations we can find this 22.5% which should be in the ratio 1:3 so the other one is 7.5%
S = 42.5 + 7.5
S = 50%
Here to find the solution, do the second thing first and then go to the first thing
The second one is mixed in the ratio 1 : 1 so that the final thing should be bang in the middle
The area of a rectangle and the square of its perimeter are in the ratio 1 : 25. Then the lengths of the shorter and longer sides of the rectangle are in the ratio
Video Explanation
Explanatory Answer
The smallest integer n for which 4n ＞ 1719 holds, is closest to
Video Explanation
Explanatory Answer
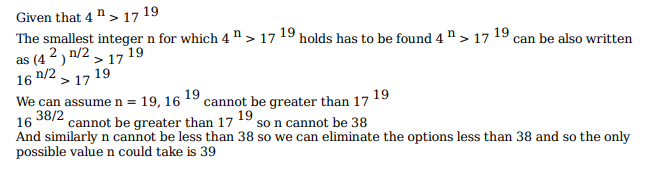
The smallest integer n such that n3 - 11n2 + 32n - 28 ＞ 0 is
Video Explanation
Explanatory Answer
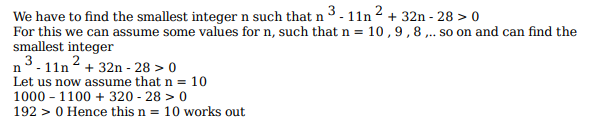
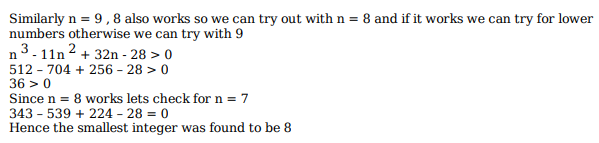
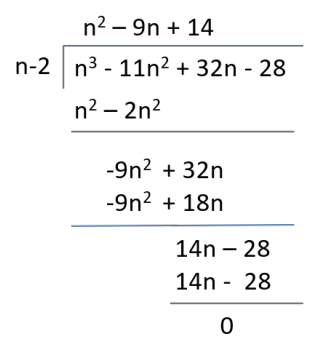
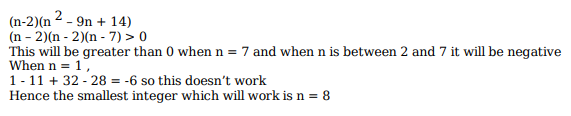
A parallelogram ABCD has area 48 sqcm. If the length of CD is 8 cm and that of AD is s cm, then which one of the following is necessarily true?
Video Explanation
Explanatory Answer
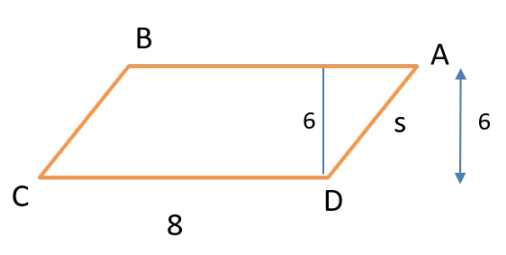
Given that a parallelogram ABCD has an area 48 sq cm. The length of CD is 8 cm and that of AD is s cm
Since the area given here is 48 sq.cm the height can be found as 6 sq.cm
We have to find which of the following options is necessarily true
Since this (shaded portion) is a right angled triangle with 6 as its height and s as its hypotenuse so we can assure that s ≥ 6 and s cannot be less than 6 so we can eliminate the other options.
By this we can come to the conclusion that s ≥ 6
The value of the sum 7 x 11 + 11 x 15 + 15 x 19 + . + 95 x 99 is
Video Explanation
Explanatory Answer
On a long stretch of east-west road, A and B are two points such that B is 350 km west of A. One car starts from A and another from B at the same time. If they move towards each other, then they meet after 1 hour. If they both move towards east, then they meet in 7 hrs. The difference between their speeds, in km per hour, is
Video Explanation
Explanatory Answer
Given that A and B are two points such that B is 350 km west of A. One car starts from A and
another from B at the same time.
They meet after one hour or their relative velocity is (a + b)km/hr and their relative distance is
350kms
a + b = 350 which is equal to 350 kms/hr
If they both move towards east, then they meet in 7 hrs.
We have to find the difference between their speeds, in km per hour. B is catching upon A, so once
again their relative distance will be 350kms.
Relative speed = b – a , B travels faster so that it can meet a.
⟹ 350/ 𝑏−𝑎 = 7
⟹ b - a = 50 km/hr
We know that b + a = 350 km/hr
By this we can find the values of a and b but they have asked for only the difference between their
speeds which is b – a = 50km/hr
If the sum of squares of two numbers is 97, then which one of the following cannot be their product?
Video Explanation
A jar contains a mixture of 175 ml water and 700 ml alcohol. Gopal takes out 10% of the mixture and substitutes it by water of the same amount. The process is repeated once again. The percentage of water in the mixture is now
Video Explanation
Explanatory Answer
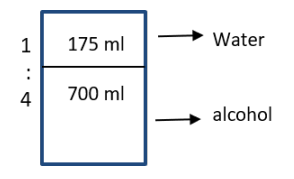
Given that a jar contains a mixture of 175 ml water and 700 ml alcohol.
It is given that 10% of the mixture is removed and it is substituted by water of the same amount
and the process is repeated once again
Now we have to find the percentage of water in the mixture.
Since the mixture is removed and substituted with water, we can deal with alcohol and the second
step we can find how much amount of alcohol is retained and not about how much amount of
alcohol is removed
As 10% of alcohol is removed, 90% of alcohol is retained
So alcohol remaining = 700 × 90% × 90%
⟹ 700 × 0.9 × 0.9 = 567
We totally have 875 ml overall mixture and of this 567 ml is alcohol.
Remaining 875 – 567 = 308 is the amount of water.
We have to find the percentage of water in the mixture i.e. 308/875
Approximately 308 is 30% of 1000 so by this we know that 308 is more than 30%
Hence 35.2% is the percentage of water in the given mixture.
Points A and B are 150 km apart. Cars 1 and 2 travel from A to B, but car 2 starts from A when car 1 is already 20 km away from A. Each car travels at a speed of 100 kmph for the first 50 km, at 50 kmph for the next 50 km, and at 25 kmph for the last 50 km. The distance, in km, between car 2 and B when car 1 reaches B is
Video Explanation
Explanatory Answer
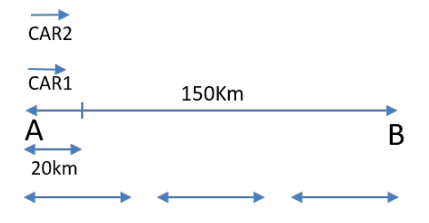
Given that points A and B are 150 km apart.
Cars 1 and 2 travel from A to B, but car 2 starts from A when car 1 is already 20 km away from A.
Each car travels at a speed of 100 kmph for the first 50 km, at 50 kmph for the next 50 km, and at
25 kmph for the last 50 km.
The Car 1 is 20km away from A and it travels at 100kmph and the time taken is 20/100 = 1/5hr
So car 2 is 12 minutes behind car 1.
We have to find the distance in km, between car 2 and B when car 1 reaches B.
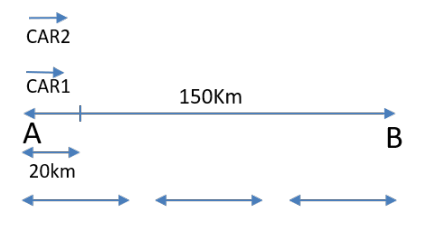
If car 1 reaches B, car 2 will take 12 minutes to reach b.
Distance between car 2 and B is 25 × 1/5 = 5 kms
The distance, in km, between car 2 and B when car 1 reaches B is 5 kms
A tank is emptied everyday at a fixed time point. Immediately thereafter, either pump A or pump B or both start working until the tank is full. On Monday, A alone completed filling the tank at 8 pm. On Tuesday, B alone completed filling the tank at 6 pm. On Wednesday, A alone worked till 5 pm, and then B worked alone from 5 pm to 7 pm, to fill the tank. At what time was the tank filled on Thursday if both pumps were used simultaneously all along?
Video Explanation
Explanatory Answer
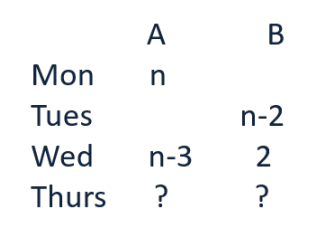
Given that a tank is emptied everyday at a fixed time point and after that either pump A or B or
both start working to fill the tank.
On Monday, A alone completed filling the tank at 8 pm.
On Tuesday, B alone completed filling the tank at 6 pm.
On Wednesday, A alone worked till 5 pm, and then B worked alone from 5 pm to 7 pm, to fill the
tank.
We have to find at what time will the tank filled on thursday if both were used simultaneously all
along
A is doing 3 hours less work on Wednesday which B completes in 2 hours therefore B = 1.5 A
⟹ (n)/(n-2) = 3/2
⟹ 2n = 3n – 6
⟹ n = 6
A takes 6 hours and B takes 4 hours or the tank is closed at 2 pm
When they are together open
⟹ 1/6 + 1/4 = (2+3)/12
⟹ Rate is 5/12 or they can fill the entire tank in 12/5 hours which is 2 hours 24 minutes
Starting from 2 pm, they fill the entire tank by 4:24 pm
Here the break through is figuring out the ratio or efficiency of the rate at which they fill is B : A
= 3 : 2
The conventional way is
⟹ (n−3)/n + 2/(n-2) = 1
n-3 hours at the rate of 1/𝑛 per hour
2 hours at the rate 1/(𝑛−2) per hour
Solving this we get n = 6 and we can find solution using this n.
Ramesh and Ganesh can together complete a work in 16 days. After seven days of working together, Ramesh got sick and his efficiency fell by 30%. As a result, they completed the work in 17 days instead of 16 days. If Ganesh had worked alone after Ramesh got sick, in how many days would he have completed the remaining work?
Video Explanation
Explanatory Answer
Given that Ramesh and Ganesh can together complete a work in 16 days
After seven days of working together, Ramesh got sick and his efficiency fell by 30%.
To the original schedule of 16 days there are 9 more days and in this 9 days Ramesh fall short by 9
× 0.3x = 2.7x where x is the work done by Ramesh in a day
So the change = 2.7x (i.e. the gap because of drop in efficiency)
This 2.7x is compensated by one day of Ganesh + another 0.7 of Ramesh
2.7x = G + 0.7 x (Because the last day also he works with reduced efficiency)
2x = G
Hence Ganesh works twice as that of Ramesh.
If Ganesh had worked alone after Ramesh got sick means in the 7 days Ramesh would have
completed 7/48 of the task
Ganesh on each day can do 1/24 of the task
Task to be completed by Ganesh is 41/48 completely
One day he can complete 1/24 so 41/48 × 24/1 = 20.5 days
If Ganesh had worked alone after Ramesh got sick, the no.of days needed for completing the
remaining work is 20.5 – 7 = 13.5 days
If a and b are integers such that 2x2 − ax + 2 ＞ 0 and x2 − bx + 8 ≥ 0 for all real numbers x, then the largest possible value of 2a − 6b is
Video Explanation
Explanatory Answer
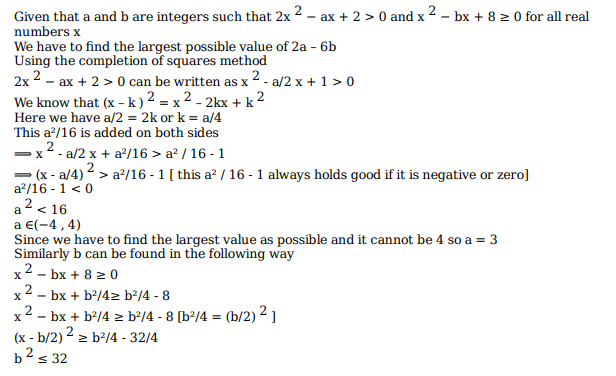
The scores of Amal and Bimal in an examination are in the ratio 11 : 14. After an appeal, their scores increase by the same amount and their new scores are in the ratio 47 : 56. The ratio of Bimal’s new score to that of his original score is
Video Explanation
Explanatory Answer
A triangle ABC has area 32 sq units and its side BC, of length 8 units, lies on the line x = 4. Then the shortest possible distance between A and the point (0,0) is
Video Explanation
Explanatory Answer
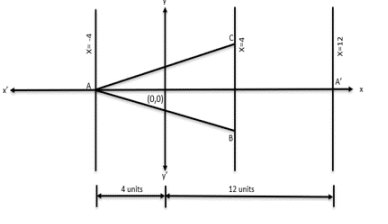
Given Area (△ABC) = 32 sq units and one of the length BC = 8 units on the line x = 4
Let us draw a graph and plot the given values.
We know that area of the Triangle = 1/2 × base × height considering BC as the base,
area of the Triangle = 1/2 × 8 × height = 32
Height = (32×2) / 8 = 64/8 = 8 units
Since the base lies on x = 4 and has a vertical height is of length = 8 units, A can either lie on the
line x = 12 or on x = - 4
However, since we need to find the shortest possible distance between A and the origin, A should
lie on the line x = - 4
So, shortest possible distance to A from the point (0,0) = 4 units
How many two-digit numbers, with a non-zero digit in the units place, are there which are more than thrice the number formed by interchanging the positions of its digits?
Video Explanation
Gopal borrows Rs. X from Ankit at 8% annual interest. He then adds Rs. Y of his own money and lends Rs. X+Y to Ishan at 10% annual interest. At the end of the year, after returning Ankit’s dues, the net interest retained by Gopal is the same as that accrued to Ankit. On the other hand, had Gopal lent Rs. X+2Y to Ishan at 10%, then the net interest retained by him would have increased by Rs. 150. If all interests are compounded annually, then find the value of X + Y.
Video Explanation
Explanatory Answer
Let us draw a flow diagram to understand the transaction
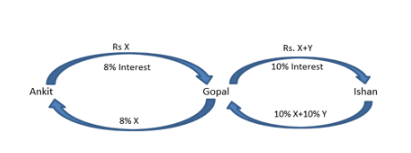
Gopal receives 10% of X + Y from Ishan as interest, from which he pays the interest of 8% of X to
Ankit
So, Gopal would gain 2% of X + 10% of Y as interest and Ankit would gain 8% Interest
Its given that both the interest values are same, so 2% X + 10% Y = 8% X
3% X = 5% Y --- (1)
Its also given that, if Gopal lend Rs. X + 2Y to Ishan at 10% interest, the net interest retained
would increase by Rs. 150
So, the increase in 10% Y accounts for Rs. 150
10% Y = 150 => Y = Rs. 1500
Substituting the value in (1), we get 5 × 1500 = 3 × X => X = 2500
So, X + Y = 2500 + 1500 = Rs 4000
Video Explanation
Let f(x)=max{5x, 52 - 2x2}, where x is any positive real number.Then the minimum possible value of f(x) is
Video Explanation
Explanatory Answer
For two sets A and B, let AΔB denote the set of elements which belong to A or B but not both. If P = {1,2,3,4}, Q = {2,3,5,6,}, R = {1,3,7,8,9}, S = {2,4,9,10}, then the number of elements in (PΔQ)Δ(RΔS) is
Video Explanation
Explanatory Answer
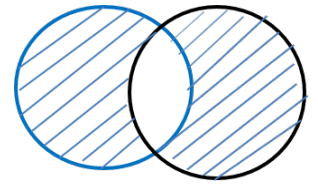
Given that for two sets A and B, A △ B denote the set of elements which belong to A or B but not
both.
This is the diagram which represents A △ B ,the set of elements which belongs to A or B i.e. A
intersection B subtracted from A union B
We have to find the number of elements in (P △ Q) △ (R △ S) if
P = {1 , 2 , 3 , 4} Q = {2 , 3 , 5 , 6 ,} R = {1 , 3 , 7 , 8 , 9} S = {2 , 4 , 9 , 10}
For P △ Q, we have to find the elements which do not to belong to both P and Q such that
(P △ Q) = {1, 4 , 5 , 6}
(R △ S) = {1 , 2 , 3 , 4 , 7 , 8 , 10}
(P △ Q) △ (R △ S) = {2 , 3 , 5 , 7 , 6 , 8 , 10}
The number of elements (P △ Q) △ (R △ S) is 7
If A = {62n - 35n - 1: n = 1,2,3,.} and B = {35(n-1) : n = 1,2,3,.} then which of the following is true?
Video Explanation
Explanatory Answer
The strength of a salt solution is p% if 100 ml of the solution contains p grams of salt. If three salt solutions A, B, C are mixed in the proportion 1 : 2 : 3, then the resulting solution has strength 20%. If instead the proportion is 3 : 2 : 1, then the resulting solution has strength 30%. A fourth solution, D, is produced by mixing B and C in the ratio 2 : 7. The ratio of the strength of D to that of A is
Video Explanation
Video Explanation
Explanatory Answer
College Accreditation
An agency entrusted to accredit colleges looks at four parameters: faculty quality (F), reputation (R), placement quality (P), and infrastructure (I). The four parameters are used to arrive at an overall score, which the agency uses to give an accreditation to the colleges. In each parameter, there are five possible letter grades given, each carrying certain points: A (50 points), B (40 points), C (30 points), D (20 points), and F (0 points). The overall score for a college is the weighted sum of the points scored in the four parameters. The weights of the parameters are 0.1, 0.2, 0.3 and 0.4 in some order, but the order is not disclosed.
Accreditation is awarded based on the following scheme:
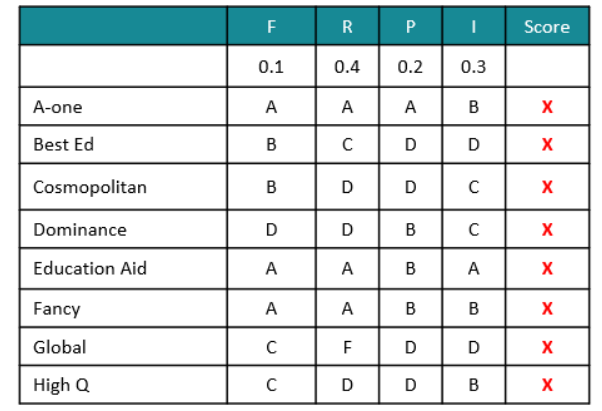
Eight colleges apply for accreditation, and receive the following grades in the four parameters (F, R, P, and I):
It is further known that in terms of overall scores:
1. High Q is better than Best Ed.
2. Best Ed is better than Cosmopolitan.
3. Education Aid is better than A-one.
What is the weight of the faculty quality parameter?
Video Explanation
Explanatory Answer
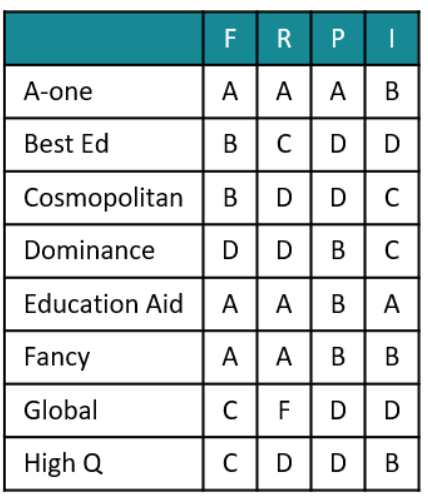
Let us look at this one at a time. Statement 1 does not give us clear comparables, let us start with
statement 2.
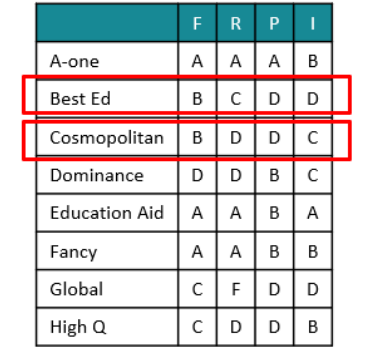
Ratings in F and P are identical, so we can infer that Weightage for R > Weightage for I.
R > I.
Now, let us move to statement 3.
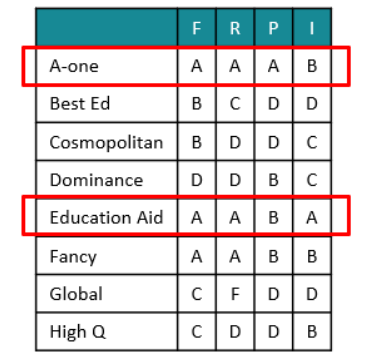
Ratings in F and R are identical, so we can infer that Weightage for I > Weightage for P.
I > P.
Now, let us combine these two inferences – we get R > I > P.
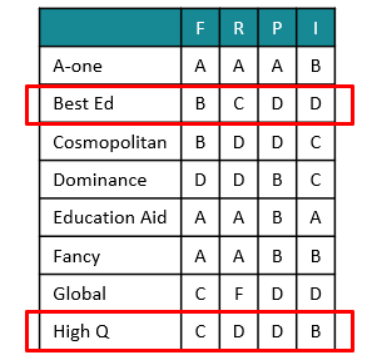
Now, let us look at Statement 1.
Scores in P are identical. Best Ed has gotten better scores in F and R and a worse score in I.
Further Best Ed has gotten better score by one grade in F and R, but worse score by two grades
in I.
So, Best Ed’s better score in two grades is more than offset by High Q’s better score in one
parameter.
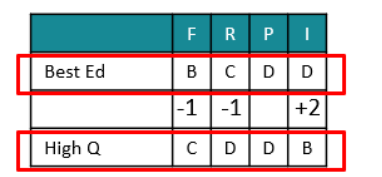
High Q is -1 in F and R, but +2 in I. We know that weightage given for R > I.
So, the -1 in R has a great impact, so the -1 in F should have much lower impact.
In other words, weightage for F should be small and that for I should be high.
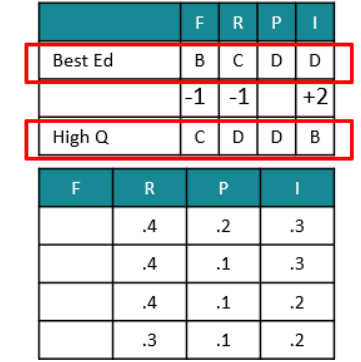
Let us account for R > I > P first.
Of the 4 possibilities, we can easily eliminate the last one as F cannot be 0.4.
F cannot be 0.3 either, so the 3rd one can also be eliminated.
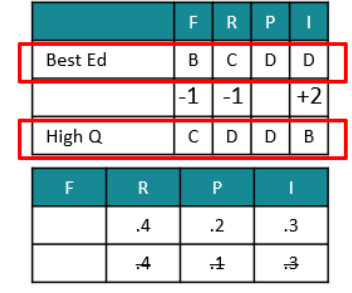
Even if F were 0.2, High Q would not be higher than Best End – the two scores would be equal.
So, the weightages have to be F = 0.1, R = 0.4, P = 0.2 and I = 0.3.
So, the weightages are as follows.
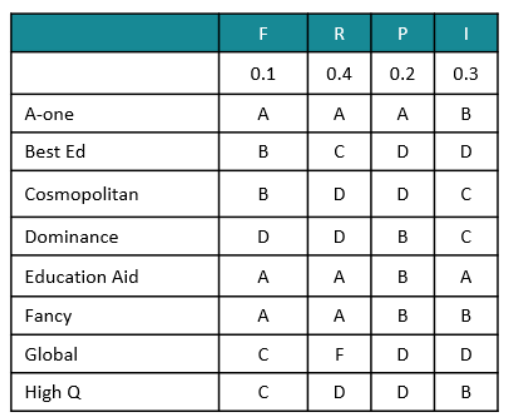
From the above table, we can see that the weight of the faculty quality parameter is 0.1.
How many colleges receive the accreditation of AAA? [TITA]
Video Explanation
Explanatory Answer
Let us look at this one at a time. Statement 1 does not give us clear comparables, let us start with
statement 2.
Ratings in F and P are identical, so we can infer that Weightage for R > Weightage for I.
R > I.
Now, let us move to statement 3.
Ratings in F and R are identical, so we can infer that Weightage for I > Weightage for P.
I > P.
Now, let us combine these two inferences – we get R > I > P.
Now, let us look at Statement 1.
Scores in P are identical. Best Ed has gotten better scores in F and R and a worse score in I.
Further Best Ed has gotten better score by one grade in F and R, but worse score by two grades
in I.
So, Best Ed’s better score in two grades is more than offset by High Q’s better score in one
parameter.
High Q is -1 in F and R, but +2 in I. We know that weightage given for R > I.
So, the -1 in R has a great impact, so the -1 in F should have much lower impact.
In other words, weightage for F should be small and that for I should be high.
Let us account for R > I > P first.
Of the 4 possibilities, we can easily eliminate the last one as F cannot be 0.4.
F cannot be 0.3 either, so the 3rd one can also be eliminated.
Even if F were 0.2, High Q would not be higher than Best End – the two scores would be equal.
So, the weightages have to be F = 0.1, R = 0.4, P = 0.2 and I = 0.3.
So, the weightages are as follows.
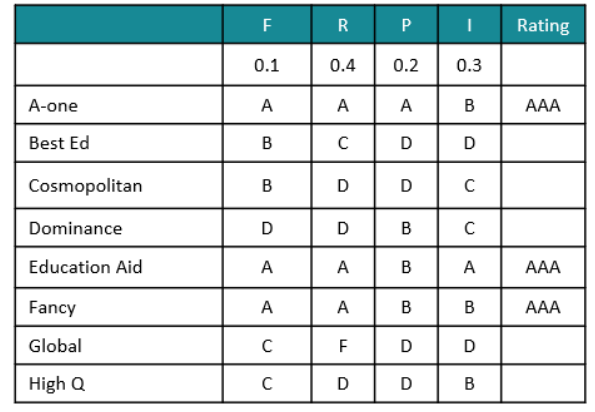
From the above table, we can see that the number of colleges which received AAA accredditation
is 3.
What is the highest overall score among the eight colleges? [TITA]
Video Explanation
Explanatory Answer
Let us look at this one at a time. Statement 1 does not give us clear comparables, let us start with
statement 2.
Ratings in F and P are identical, so we can infer that Weightage for R > Weightage for I.
R > I.
Now, let us move to statement 3.
Ratings in F and R are identical, so we can infer that Weightage for I > Weightage for P.
I > P.
Now, let us combine these two inferences – we get R > I > P.
Now, let us look at Statement 1.
Scores in P are identical. Best Ed has gotten better scores in F and R and a worse score in I.
Further Best Ed has gotten better score by one grade in F and R, but worse score by two grades
in I.
So, Best Ed’s better score in two grades is more than offset by High Q’s better score in one
parameter.
High Q is -1 in F and R, but +2 in I. We know that weightage given for R > I.
So, the -1 in R has a great impact, so the -1 in F should have much lower impact.
In other words, weightage for F should be small and that for I should be high.
Let us account for R > I > P first.
Of the 4 possibilities, we can easily eliminate the last one as F cannot be 0.4.
F cannot be 0.3 either, so the 3rd one can also be eliminated.
Even if F were 0.2, High Q would not be higher than Best End – the two scores would be equal.
So, the weightages have to be F = 0.1, R = 0.4, P = 0.2 and I = 0.3.
So, the weightages are as follows.
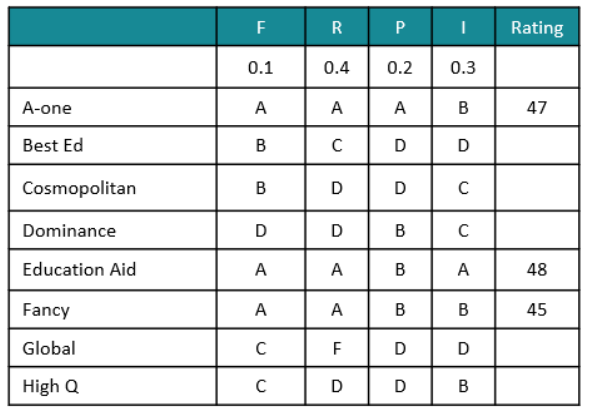
From the above table, we can see that the highest overall score among the eight colleges is 48.
How many colleges have overall scores between 31 and 40, both inclusive?
Video Explanation
Explanatory Answer
Let us look at this one at a time. Statement 1 does not give us clear comparables, let us start with
statement 2.
Ratings in F and P are identical, so we can infer that Weightage for R > Weightage for I.
R > I.
Now, let us move to statement 3.
Ratings in F and R are identical, so we can infer that Weightage for I > Weightage for P.
I > P.
Now, let us combine these two inferences – we get R > I > P.
Now, let us look at Statement 1.
Scores in P are identical. Best Ed has gotten better scores in F and R and a worse score in I.
Further Best Ed has gotten better score by one grade in F and R, but worse score by two grades
in I.
So, Best Ed’s better score in two grades is more than offset by High Q’s better score in one
parameter.
High Q is -1 in F and R, but +2 in I. We know that weightage given for R > I.
So, the -1 in R has a great impact, so the -1 in F should have much lower impact.
In other words, weightage for F should be small and that for I should be high.
Let us account for R > I > P first.
Of the 4 possibilities, we can easily eliminate the last one as F cannot be 0.4.
F cannot be 0.3 either, so the 3rd one can also be eliminated.
Even if F were 0.2, High Q would not be higher than Best End – the two scores would be equal.
So, the weightages have to be F = 0.1, R = 0.4, P = 0.2 and I = 0.3.
So, the weightages are as follows.
From the above table, we can see that the number of colleges which have overall scores between
31 and 40 both inclusive is 0.
Smartphones
There are only four brands of entry level smartphones called Azra, Bysi, Cxqi, and Dipq in a country. Details about their market share, unit selling price, and profitability (defined as the profit as a percentage of the revenue) for the year 2016 are given in the table below:
In 2017, sales volume of entry level smartphones grew by 40% as compared to that in 2016. Cxqi offered a 40% discount on its unit selling price in 2017, which resulted in a 15% increase in its market share. Each of the other three brands lost 5% market share. However, the profitability of Cxqi came down to half of its value in 2016. The unit selling prices of the other three brands and their profitability values remained the same in 2017 as they were in 2016.
The brand that had the highest revenue in 2016 is:
Video Explanation
Explanatory Answer
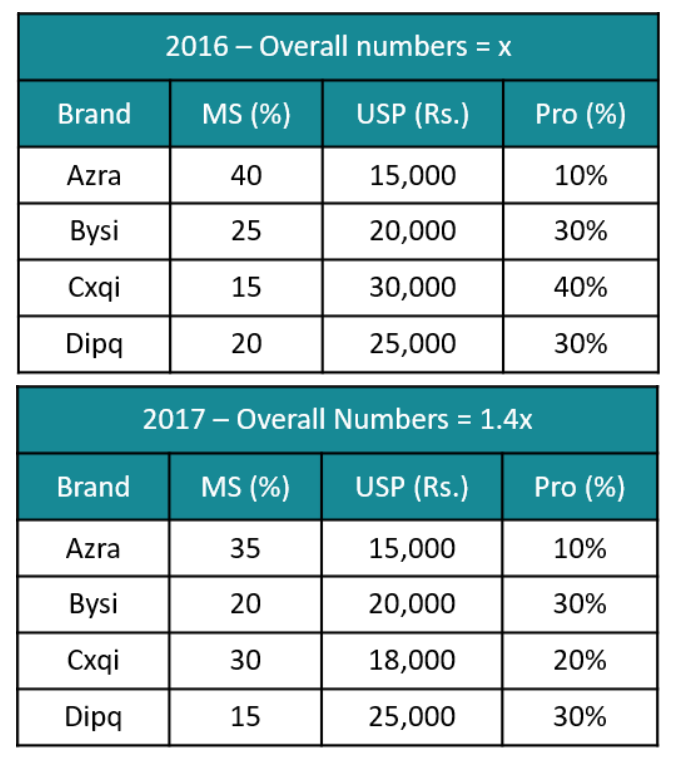
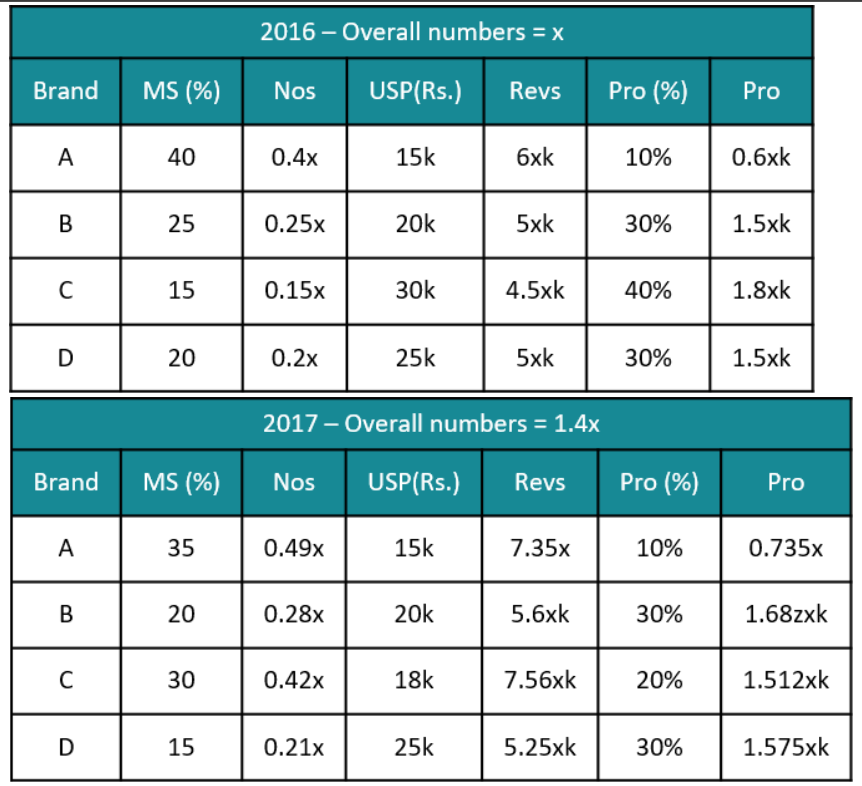
From the above table, we can see that Azra had the highest revenue in 2016.
The brand that had the highest profit in 2016 is:
Video Explanation
Explanatory Answer
From the above table, we can see that Cxqi had the highest profit in 2016.
The brand that had the highest profit in 2017 is:
Video Explanation
Explanatory Answer
From the above table, we can see that Bysi had the highest profit in 2017.
The complete list of brands whose profits went up in 2017 from 2016 is:
Video Explanation
Explanatory Answer
From the above table, we can see that the profits of Azra, Bysi, Dipq went up in 2017 from 2016.
Fun Sports Club
Fun Sports (FS) provides training in three sports - Gilli-danda (G), Kho-Kho (K), and Ludo (L). Currently it has an enrollment of 39 students each of whom is enrolled in at least one of the three sports. The following details are known:
1. The number of students enrolled only in L is double the number of students enrolled in all the three sports.
2. There are a total of 17 students enrolled in G.
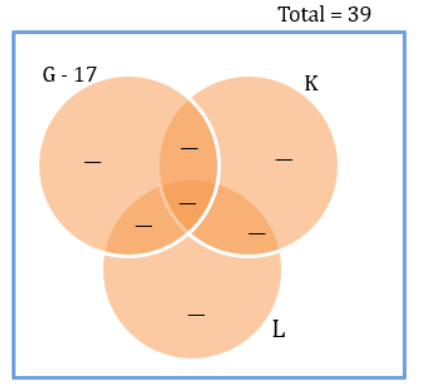
3. The number of students enrolled only in G is one less than the number of students enrolled only in L.
4. The number of students enrolled only in K is equal to the number of students who are enrolled in both K and L.
5. The maximum student enrollment is in L.
6. Ten students enrolled in G are also enrolled in at least one more sport.
What is the minimum number of students enrolled in both G and L but not in K? [TITA]
Video Explanation
Explanatory Answer
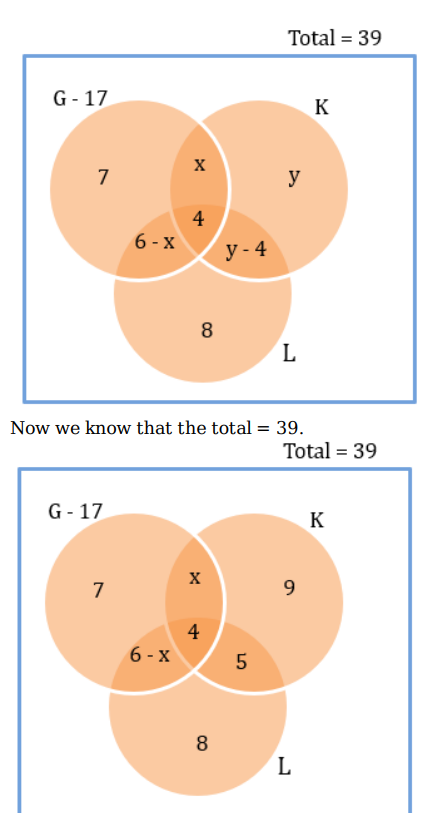
From condition 3, we get the above diagram
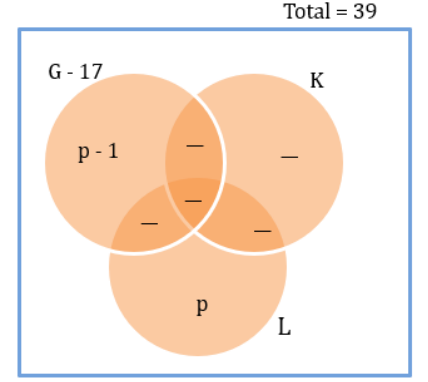
Condition 6 tells us p – 1 = 7. G-total = 17. G and something else = 10. G-only should be 7.
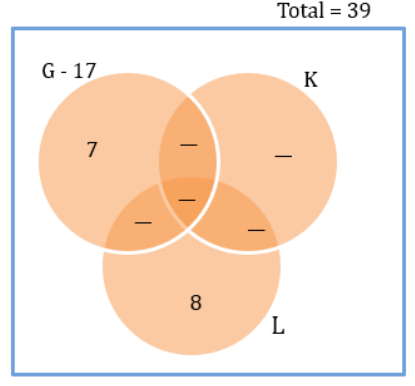
From condition 1, we get the above diagram.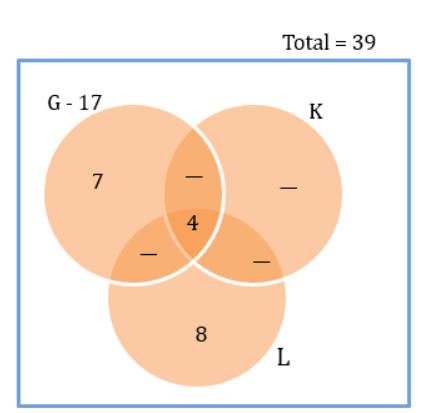 
From condition 6, we can get the diagram.
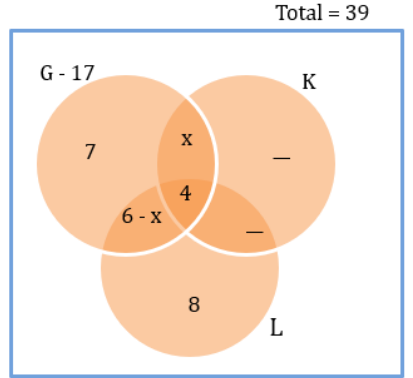
From condition 4, we get the above diagram.
From condition 5,
Number of students in L = 4 + 5 + 8 + 6 – x = 23- x.
Number of students in K = 4 + 5 + 9 + x = 18 + x.
We know that 23 – x > 18 + x.
5 > 2x. x < 5252.
x could be 0, 1 or 2.
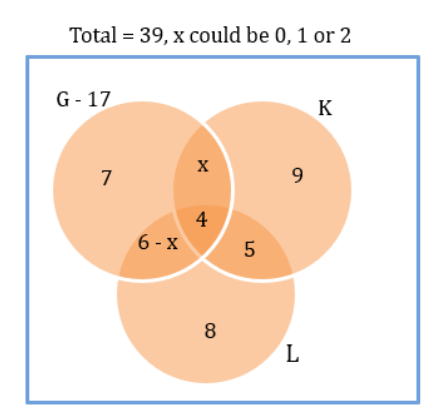
For 6 - x to be minimum, x should be maximum i.e. x should take 2.
Therefore the minimum number of students enrolled in both G and L but not in K is 4.
If the numbers of students enrolled in K and L are in the ratio 19:22, then what is the number of students enrolled in L?
Video Explanation
Explanatory Answer
From condition 3, we get the above diagram
Condition 6 tells us p – 1 = 7. G-total = 17. G and something else = 10. G-only should be 7.
From condition 1, we get the above diagram.
From condition 6, we can get the diagram.
From condition 4, we get the above diagram.
From condition 5,
Number of students in L = 4 + 5 + 8 + 6 – x = 23- x.
Number of students in K = 4 + 5 + 9 + x = 18 + x.
We know that 23 – x > 18 + x.
5 > 2x. x < 5252.
x could be 0, 1 or 2.
Number of students in L = 4 + 5 + 8 + 6 – x = 23- x.
Number of students in K = 4 + 5 + 9 + x = 18 + x.
Ratio of K : L = 19 : 22. or, x = 1.
Or, number of students in L = 22.
Due to academic pressure, students who were enrolled in all three sports were asked to withdraw from one of the three sports. After the withdrawal, the number of students enrolled in G was six less than the number of students enrolled in L, while the number of students enrolled in K went down by one.After the withdrawal, how many students were enrolled in both G and K? [TITA]
Video Explanation
Explanatory Answer
From condition 3, we get the above diagram
Condition 6 tells us p – 1 = 7. G-total = 17. G and something else = 10. G-only should be 7.
From condition 1, we get the above diagram.
From condition 6, we can get the diagram.
From condition 4, we get the above diagram.
From condition 5,
Number of students in L = 4 + 5 + 8 + 6 – x = 23- x.
Number of students in K = 4 + 5 + 9 + x = 18 + x.
We know that 23 – x > 18 + x.
5 > 2x. x < 5252.
x could be 0, 1 or 2.
We know that G ∩ K ∩ L = 0. We know that the 4 that were originally here are distributed among
the three regions currently having x, 6 – x and 5.
We know that one student leaves K. So, this student should have gone to the region (G and L but
not K).
Or, (G and L but not K) will now read 7 – x.
The other three should have opted out of one or the other of G and L. Let us assume m students
left G, 3 – m should have left L.
Let us rejig the diagram.
Total number of students in G = 17 – m.
Total number of students in L = 20 + m – x.
20 + m – x – (17 – m) = 3 + 2m – x = 6.
2m – x = 3. x can only take values 0, 1 and 2. 2m = 3 + x.
Or, x has to be 1. m has to be 2.
Both G and K = 3 + x – m. 3 + 1 -2 = 2.
Due to academic pressure, students who were enrolled in all three sports were asked to withdraw from one of the three sports. After the withdrawal, the number of students enrolled in G was six less than the number of students enrolled in L, while the number of students enrolled in K went down by one.After the withdrawal, how many students were enrolled in both G and L?
Video Explanation
Explanatory Answer
From condition 3, we get the above diagram
Condition 6 tells us p – 1 = 7. G-total = 17. G and something else = 10. G-only should be 7.
From condition 1, we get the above diagram.
From condition 6, we can get the diagram.
From condition 4, we get the above diagram.
From condition 5,
Number of students in L = 4 + 5 + 8 + 6 – x = 23- x.
Number of students in K = 4 + 5 + 9 + x = 18 + x.
We know that 23 – x > 18 + x.
5 > 2x. x < 5252.
x could be 0, 1 or 2.
The other three should have opted out of one or the other of G and L. Let us assume m students
left G, 3 – m should have left L.
Let us rejig the diagram.
Total number of students in G = 17 – m.
Total number of students in L = 20 + m – x.
20 + m – x – (17 – m) = 3 + 2m – x = 6.
2m – x = 3. x can only take values 0, 1 and 2. 2m = 3 + x.
Or, x has to be 1. m has to be 2.
Both G and L = 7 – x = 6.
Products and Companies
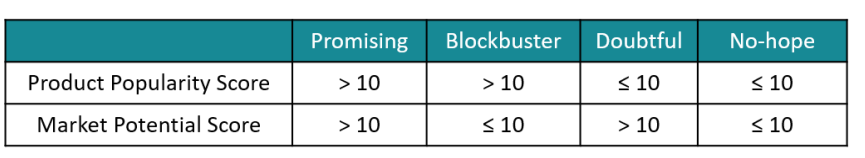
Each of the 23 boxes in the picture below represents a product manufactured by one of the following three companies: Alfa, Bravo and Charlie. The area of a box is proportional to the revenue from the corresponding product, while its centre represents the Product popularity and Market potential scores of the product (out of 20). The shadings of some of the boxes have got erased.
The companies classified their products into four categories based on a combination of scores (out of 20) on the two parameters - Product popularity and Market potential as given below:
The following facts are known:
1. Alfa and Bravo had the same number of products in the Blockbuster category.
2. Charlie had more products than Bravo but fewer products than Alfa in the No-hope category.
3. Each company had an equal number of products in the Promising category.
4. Charlie did not have any product in the Doubtful category, while Alfa had one product more than Bravo in this category.
5. Bravo had a higher revenue than Alfa from products in the Doubtful category.
6. Charlie had a higher revenue than Bravo from products in the Blockbuster category.
7. Bravo and Charlie had the same revenue from products in the No-hope category.
8. Alfa and Charlie had the same total revenue considering all products.
Considering all companies products, which product category had the highest revenue?
Video Explanation
Explanatory Answer
The companies classified their products into four categories based on a combination of scores (out
of 20) on the two parameters - Product popularity and Market potential as given below
From condition 1, Alfa and Bravo should have 2 each, Charlie should have 3.
From condition 2, Bravo = 1, Charlie = 2, Alfa = 3.
From condition 3, Alfa = Bravo = Charlie = 1.
From condition 4, Alpha = 4, Bravo = 3, Charlie = 0.
Let us capture this differently and then take it from there.
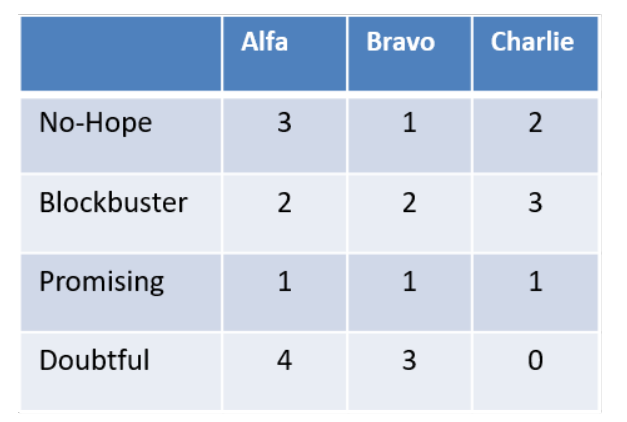
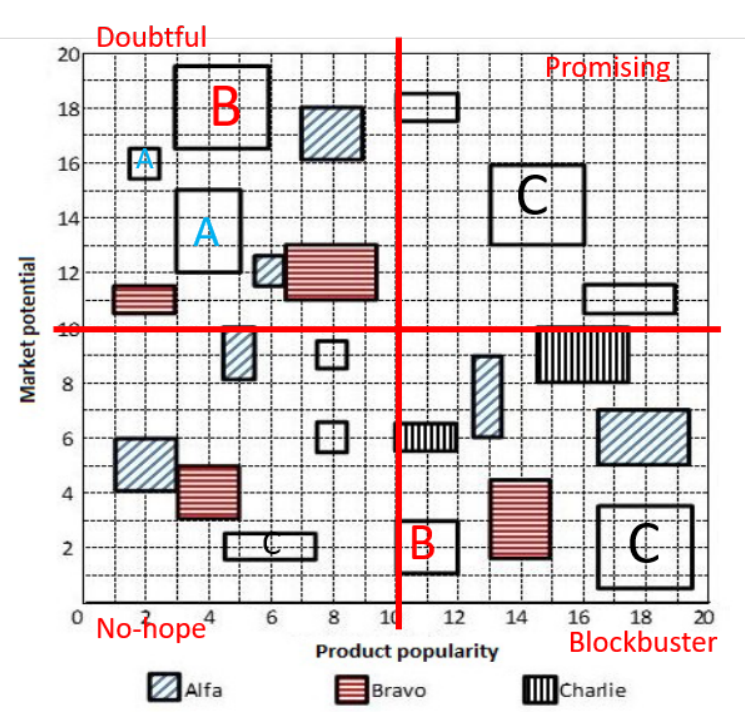
From condition 5, The big free square is Bravo.
From condition 6, The big free square is Charlie.
From condition 7, Charlie has rectangle and Square.
From condition 8, Chances are the big square in Promising is Charlie’s.
It is between Blockbuster and Doubtful. Doubtful has two small squares whereas everything in
Blockbuster is sizable. Therefore Blockbuster had the highest revenue.
Which of the following is the correct sequence of numbers of products Bravo had in No-hope, Doubtful, Promising and Blockbuster categories respectively?
Video Explanation
Explanatory Answer
The companies classified their products into four categories based on a combination of scores (out
of 20) on the two parameters - Product popularity and Market potential as given below
From condition 1, Alfa and Bravo should have 2 each, Charlie should have 3.
From condition 2, Bravo = 1, Charlie = 2, Alfa = 3.
From condition 3, Alfa = Bravo = Charlie = 1.
From condition 4, Alpha = 4, Bravo = 3, Charlie = 0.
Let us capture this differently and then take it from there.
From condition 5, The big free square is Bravo.
From condition 6, The big free square is Charlie.
From condition 7, Charlie has rectangle and Square.
From condition 8, Chances are the big square in Promising is Charlie’s.
From the table we can see that Choice B 1,3,1,2 is the correct sequence of no. of products.
Which of the following statements is NOT correct?
Video Explanation
Explanatory Answer
The companies classified their products into four categories based on a combination of scores (out
of 20) on the two parameters - Product popularity and Market potential as given below
From condition 1, Alfa and Bravo should have 2 each, Charlie should have 3.
From condition 2, Bravo = 1, Charlie = 2, Alfa = 3.
From condition 3, Alfa = Bravo = Charlie = 1.
From condition 4, Alpha = 4, Bravo = 3, Charlie = 0.
Let us capture this differently and then take it from there.
From condition 5, The big free square is Bravo.
From condition 6, The big free square is Charlie.
From condition 7, Charlie has rectangle and Square.
From condition 8, Chances are the big square in Promising is Charlie’s.
A) Alfa Block Buster revenues = 9 squares. Charlie Promising Revenues = 9 square units. This is
correct.
B) Bravo Blockbuster = 10 squares, Alfa doubtful = 12 squares.This is not correct.
C) No-Hope = 15 squares, Doubtful = 29 squares. This is correct.
D) Given in the question. This is correct.
If the smallest box on the grid is equivalent to revenue of Rs.1 crore, then what approximately was the total revenue of Bravo in Rs. crore?
Video Explanation
Explanatory Answer
The companies classified their products into four categories based on a combination of scores (out
of 20) on the two parameters - Product popularity and Market potential as given below
From condition 1, Alfa and Bravo should have 2 each, Charlie should have 3.
From condition 2, Bravo = 1, Charlie = 2, Alfa = 3.
From condition 3, Alfa = Bravo = Charlie = 1.
From condition 4, Alpha = 4, Bravo = 3, Charlie = 0.
Let us capture this differently and then take it from there.
From condition 5, The big free square is Bravo.
From condition 6, The big free square is Charlie.
From condition 7, Charlie has rectangle and Square.
From condition 8, Chances are the big square in Promising is Charlie’s.
Bravo’s revenues are from 7 companies.
In No-hope, the revenues should be Rs. 4 Crores.
In Blockbuster, the revenues should be 4 + 6 = Rs. 10 Crores.
In Promising, the revenues should be Rs. 2 Crores.(this could be 3)
In Doubtful, the revenues should be 9 + 6 + 2 = Rs. 17 Crores.
This adds up to Rs. 33 crores.
The answer choice should be C.
Amusement Park Tickets
Each visitor to an amusement park needs to buy a ticket. Tickets can be Platinum, Gold, or Economy. Visitors are classified as Old, Middle-aged, or Young. The following facts are known about visitors and ticket sales on a particular day:
1. 140 tickets were sold.
2. The number of Middle-aged visitors was twice the number of Old visitors, while the number of Young visitors was twice the number of Middle-aged visitors.
3. Young visitors bought 38 of the 55 Economy tickets that were sold, and they bought half the total number of Platinum tickets that were sold.
4. The number of Gold tickets bought by Old visitors was equal to the number of Economy tickets bought by Old visitors.
If the number of Old visitors buying Platinum tickets was equal to the number of Middle-aged visitors buying Platinum tickets, then which among the following could be the total number of Platinum tickets sold?
Video Explanation
Explanatory Answer
From second constraint,
OLD Visitors = x, MID = 2x, Young = 4x.
7x = 140 or, x = 20 , 2x = 40 , 4x = 80.
Let us fill this in. and then go to constraint 3 and 4.
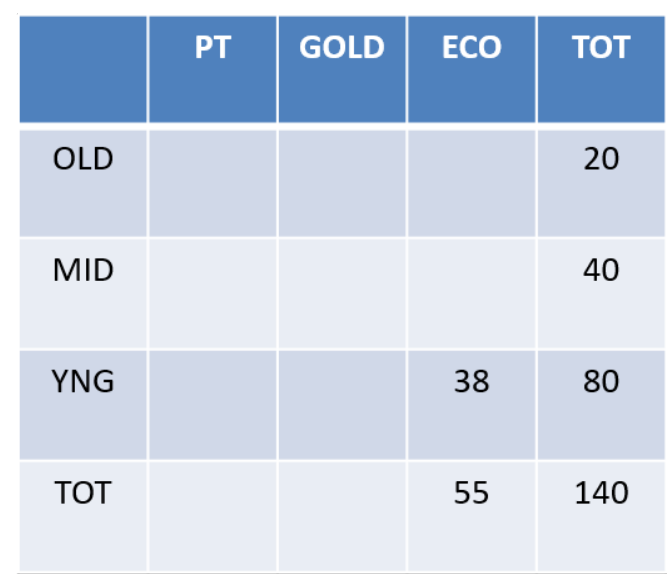
From constraint 3, Total Platinum = 2y, then YNG platinum should be y.
From constraint 4, Let us fill OLD-GOLD and OLD-ECO has z.
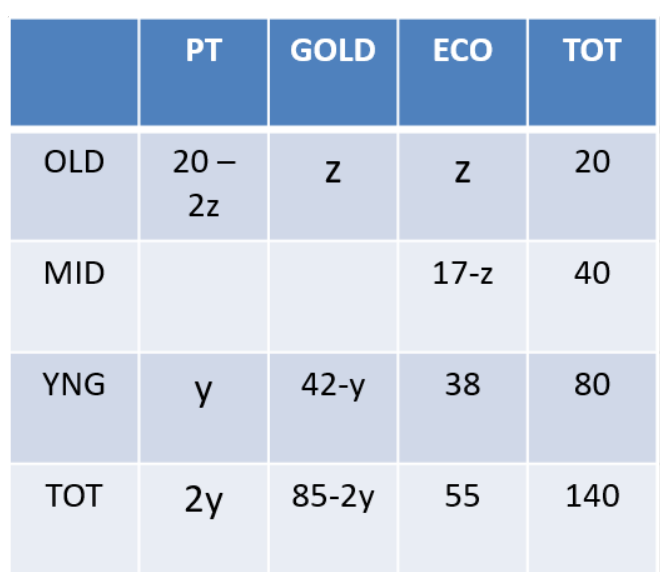
OLD-PT should be equal to MID-PT, OLD-PT and MID-PT add up to y.
So, each should be y2𝑦2. Now, we know that y2𝑦2 = 20 – 2z.
Total number of PT seats = 2y = 4 (20 – 2z) = 8 (10 – z). So, this number should be a multiple of 8.
The only possible answer is 32
If the number of Old visitors buying Gold tickets was strictly greater than the number of Young visitors buying Gold tickets, then the number Middle-aged visitors buying Gold tickets was [TITA]
Video Explanation
Explanatory Answer
From second constraint,
OLD Visitors = x, MID = 2x, Young = 4x.
7x = 140 or, x = 20 , 2x = 40 , 4x = 80.
Let us fill this in. and then go to constraint 3 and 4.
From constraint 3, Total Platinum = 2y, then YNG platinum should be y.
From constraint 4, Let us fill OLD-GOLD and OLD-ECO has z.
OLD-GOLD > YNG-GOLD.
Z > 42 – y Or, y + z > 42
We need to find MID-GOLD. This is equal to 85 – 2y – z – (42 – y) = 43 – (y + z).
We know that y + z > 42. So, MID-GOLD has to be 0.
If the number of Old visitors buying Platinum tickets was equal to the number of Middle-aged visitors buying Economy tickets, then the number of Old visitors buying Gold tickets was [TITA]
Video Explanation
Explanatory Answer
From second constraint,
OLD Visitors = x, MID = 2x, Young = 4x.
7x = 140 or, x = 20 , 2x = 40 , 4x = 80.
Let us fill this in. and then go to constraint 3 and 4.
From constraint 3, Total Platinum = 2y, then YNG platinum should be y.
From constraint 4, Let us fill OLD-GOLD and OLD-ECO has z.
OLD-PT = MID-ECO. MID-ECO = 17 – z or, OLD-PT = 17 – z.
17 – z = - 20 – 2z. Or, z = 3.
Which of the following statements MUST be FALSE?
Video Explanation
Explanatory Answer
From second constraint,
OLD Visitors = x, MID = 2x, Young = 4x.
7x = 140 or, x = 20 , 2x = 40 , 4x = 80.
Let us fill this in. and then go to constraint 3 and 4.
From constraint 3, Total Platinum = 2y, then YNG platinum should be y.
From constraint 4, Let us fill OLD-GOLD and OLD-ECO has z.
Let us go statement-by-statement and see if we can find some easy one that HAS to be FALSE.
(A) The numbers of Gold and Platinum tickets bought by Young visitors were equal.
YNG-PT = YNG-GOLD. These two should add up to 42. Or, both should be 21. Total PT should be
42. Total GOLD should be 43.
All of these appear to be possible. Let us look at the other choices and see if we can find an easier
CLEARLY FALSE statement.
Else, we will revisit this.
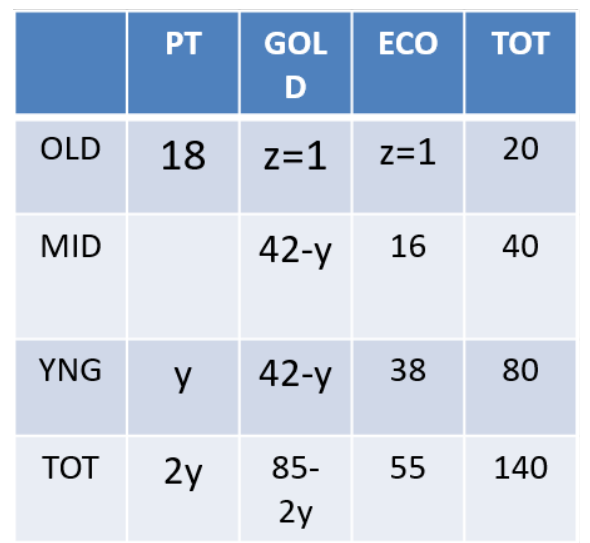
(B) The numbers of Middle-aged and Young visitors buying Gold tickets were equal.
MID-GOLD = YNG-GOLD. MID-GOLD should also be 42 – y. This tells us that z should be 1. This
also appears prima-facie possible.
Let us look at the others also before we revisit this one and completely fill the grid.
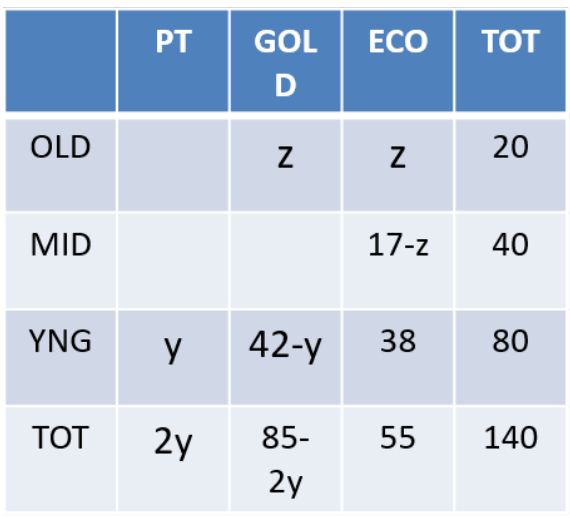
(C) The numbers of Old and Middle-aged visitors buying Economy tickets were equal.
OLD-ECO = MID-ECO.These two should add up to 17 so these two CANNOT be equal.
So, statement C is DEFINITELY FALSE.
(D) The numbers of Old and Middle-aged visitors buying Platinum tickets were equal.
OLD-PT = MID-PT. Both of these should be y2𝑦2. This also appears to be alright.
Job Interview
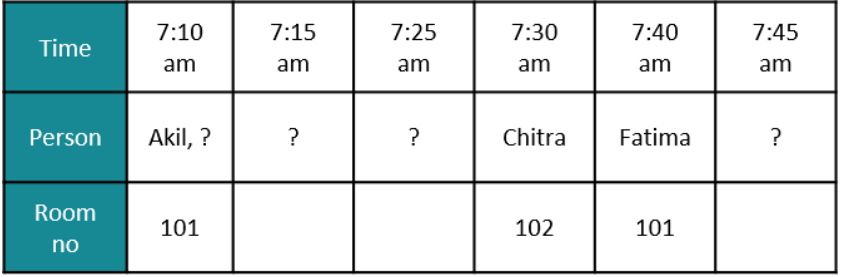
Seven candidates, Akil, Balaram, Chitra, Divya, Erina, Fatima, and Ganeshan, were invited to interview for a position. Candidates were required to reach the venue before 8 am. Immediately upon arrival, they were sent to one of three interview rooms: 101, 102, and 103. The following venue log shows the arrival times for these candidates. Some of the names have not been recorded in the log and have been marked as ‘?’.
Additionally here are some statements from the candidates:
Balaram: I was the third person to enter Room 101.
Chitra: I was the last person to enter the room I was allotted to.
Erina: I was the only person in the room I was allotted to.
Fatima: Three people including Akil were already in the room that I was allotted to when I entered it.
Ganeshan: I was one among the two candidates allotted to Room 102.
What best can be said about the room to which Divya was allotted?
Video Explanation
Explanatory Answer
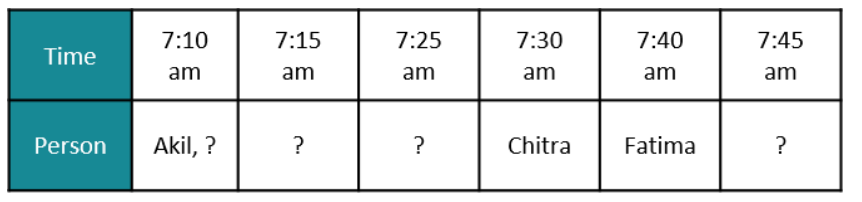
Look at the statements given by Erina and Ganeshan.
Think about the number of candidates in the rooms.
One room has only one candidate, one room has 2 candidates, so the third room should have 4
candidates.
So, number of candidates should be distributed as 4, 2, 1.
But which room has 2 candidates and which one has 4?
Room number 102 has 2 candidates. G plus one more. Balaram was the third one to enter 101, so
101 should have had at least 3 candidates.
This implies 103 has only one candidate.
Fatima is the 4th one into a room. What does this mean? Akhil should also be in the same room as
Fatima.
A, B and F go to room number 101. C should go to 102, E should go to 103.
Fatima is the 4th one into a room. Chitra is the last to enter her room.
So, the one at 7:45 should be entering a different room. Who is this?
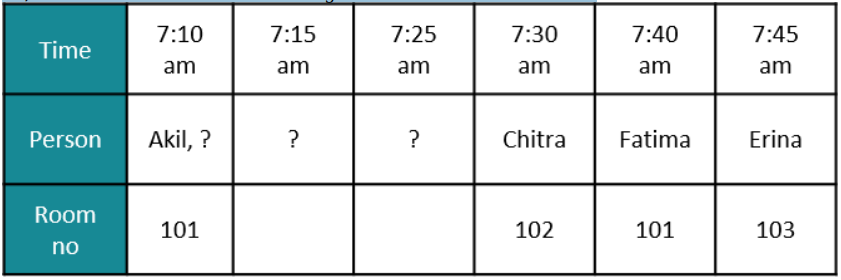
A, B and F go to room number 101. C should go to 102, E should go to 103. E should be the one
who goes at 7:45 am.
We have B, D and G remaining. B and D go into 101, G goes to 102. B comes after D as B is the
third to go into 101.
Write down the different combinations.
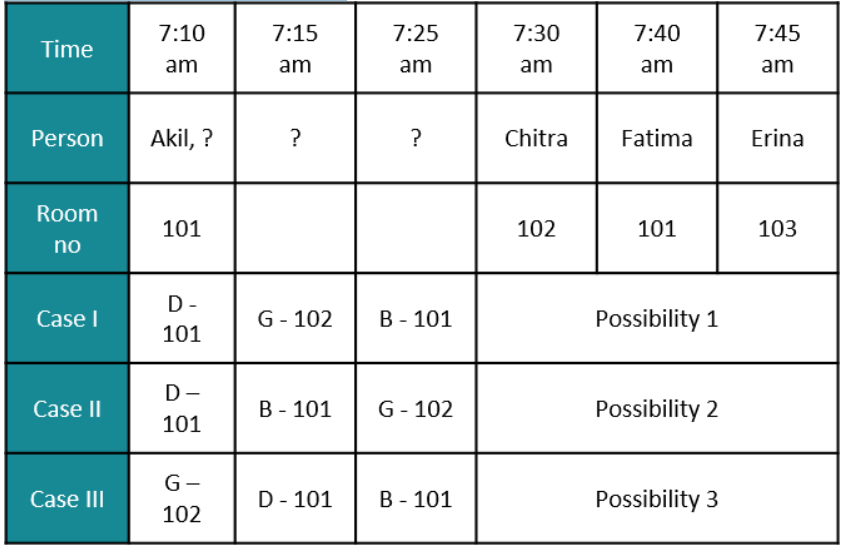
7:10, 7:15 and 7:25 could be D, B, G or D, G, B or G, D, B.
Let us move on to questions.
From the above table, we can see that Divya is definitely in room 101.
Who else was in Room 102 when Ganeshan entered?
Video Explanation
Explanatory Answer
Look at the statements given by Erina and Ganeshan.
Think about the number of candidates in the rooms.
One room has only one candidate, one room has 2 candidates, so the third room should have 4
candidates.
So, number of candidates should be distributed as 4, 2, 1.
But which room has 2 candidates and which one has 4?
Room number 102 has 2 candidates. G plus one more. Balaram was the third one to enter 101, so
101 should have had at least 3 candidates.
This implies 103 has only one candidate.
Fatima is the 4th one into a room. What does this mean? Akhil should also be in the same room as
Fatima.
A, B and F go to room number 101. C should go to 102, E should go to 103.
Fatima is the 4th one into a room. Chitra is the last to enter her room.
So, the one at 7:45 should be entering a different room. Who is this?
A, B and F go to room number 101. C should go to 102, E should go to 103. E should be the one
who goes at 7:45 am.
We have B, D and G remaining. B and D go into 101, G goes to 102. B comes after D as B is the
third to go into 101.
Write down the different combinations.
7:10, 7:15 and 7:25 could be D, B, G or D, G, B or G, D, B.
Let us move on to questions.
Ganeshan was the first to enter room 102. Therefore no one was there before Ganeshan entered
room 102.
When did Erina reach the venue?
Video Explanation
Explanatory Answer
Look at the statements given by Erina and Ganeshan.
Think about the number of candidates in the rooms.
One room has only one candidate, one room has 2 candidates, so the third room should have 4
candidates.
So, number of candidates should be distributed as 4, 2, 1.
But which room has 2 candidates and which one has 4?
Room number 102 has 2 candidates. G plus one more. Balaram was the third one to enter 101, so
101 should have had at least 3 candidates.
This implies 103 has only one candidate.
Fatima is the 4th one into a room. What does this mean? Akhil should also be in the same room as
Fatima.
A, B and F go to room number 101. C should go to 102, E should go to 103.
Fatima is the 4th one into a room. Chitra is the last to enter her room.
So, the one at 7:45 should be entering a different room. Who is this?
A, B and F go to room number 101. C should go to 102, E should go to 103. E should be the one
who goes at 7:45 am.
We have B, D and G remaining. B and D go into 101, G goes to 102. B comes after D as B is the
third to go into 101.
Write down the different combinations.
7:10, 7:15 and 7:25 could be D, B, G or D, G, B or G, D, B.
Let us move on to questions.
From the table, we can see that Erina was the last one to reach i.e. at 7:45 am.
If Ganeshan entered the venue before Divya, when did Balaram enter the venue?
Video Explanation
Explanatory Answer
Look at the statements given by Erina and Ganeshan.
Think about the number of candidates in the rooms.
One room has only one candidate, one room has 2 candidates, so the third room should have 4
candidates.
So, number of candidates should be distributed as 4, 2, 1.
But which room has 2 candidates and which one has 4?
Room number 102 has 2 candidates. G plus one more. Balaram was the third one to enter 101, so
101 should have had at least 3 candidates.
This implies 103 has only one candidate.
Fatima is the 4th one into a room. What does this mean? Akhil should also be in the same room as
Fatima.
A, B and F go to room number 101. C should go to 102, E should go to 103.
Fatima is the 4th one into a room. Chitra is the last to enter her room.
So, the one at 7:45 should be entering a different room. Who is this?
A, B and F go to room number 101. C should go to 102, E should go to 103. E should be the one
who goes at 7:45 am.
We have B, D and G remaining. B and D go into 101, G goes to 102. B comes after D as B is the
third to go into 101.
Write down the different combinations.
7:10, 7:15 and 7:25 could be D, B, G or D, G, B or G, D, B.
Let us move on to questions.
If Ganeshan entered the venue before Divya, we are looking at possibility 3 from the table.
Therefore Balaram entered the venue at 7:25 am
Will a day come when India’s poor can access government services as easily as drawing cash from an ATM? No country in the world has made accessing education or health or policing or dispute resolution as easy as an ATM, because the nature of these activities requires individuals to use their discretion in a positive way. Technology can certainly facilitate this in a variety of ways if it is seen as one part of an overall approach, but the evidence so far in education, for instance, is that just adding computers alone doesn’t make education any better.
The dangerous illusion of technology is that it can create stronger, top down accountability of service providers in implementation-intensive services within existing public sector organisations. One notion is that electronic management information systems (EMIS) keep better track of inputs and those aspects of personnel that are ‘EMIS visible’ can lead to better services. A recent study examined attempts to increase attendance of Auxiliary Nurse Midwife (ANMs) at clinics in Rajasthan, which involved high-tech time clocks to monitor attendance. The study’s title says it all: Band-Aids on a Corpse. E-governance can be just as bad as any other governance when the real issue is people and their motivation.
For services to improve, the people providing the services have to want to do a better job with the skills they have. A study of medical care in Delhi found that even though providers, in the public sector had much better skills than private sector providers their provision of care in actual practice was much worse.
In implementation-intensive services the key to success is face-to-face interactions between a teacher, a nurse, a policeman, an extension agent and a citizen. This relationship is about power. Amartya Sen’s report on education in West Bengal had a supremely telling anecdote in which the villagers forced the teacher to attend school, but then, when the parents went off to work, the teacher did not teach, but forced the children to massage his feet. As long as the system empowers providers over citizens, technology is irrelevant.
The answer to successfully providing basic services is to create systems that provide both autonomy and accountability. In basic education for instance, the answer to poor teaching is not controlling teachers more. The key is to hire teachers who want to teach and let them teach, expressing their professionalism and vocation as a teacher through autonomy in the classroom. This autonomy has to be matched with accountability for results—not just narrowly measured through test scores, but broadly for the quality of the education they provide.
A recent study in Uttar Pradesh showed that if, somehow, all civil service teachers could be replaced with contract teachers, the state could save a billion dollars a year in revenue and double student learning. Just the additional autonomy and accountability of contracts through local groups—even without complementary system changes in information and empowerment—led to that much improvement. The first step to being part of the solution is to create performance information accessible to those outside of the government.
In the context of the passage, we can infer that the title “Band Aids on a Corpse” (in paragraph 2) suggests that:
Video Explanation
Explanatory Answer
According to the author, service delivery in Indian education can be improved in all of the following ways EXCEPT through:
Video Explanation
Explanatory Answer
Which of the following, IF TRUE, would undermine the passage’s main argument?
Video Explanation
Explanatory Answer
The author questions the use of monitoring systems in services that involve face-to-face interaction between service providers and clients because such systems:
Video Explanation
Explanatory Answer
The main purpose of the passage is to:
Video Explanation
Explanatory Answer
Letter Codes
According to a coding scheme the sentence,
Peacock is designated as the national bird of India is coded as 5688999 35 1135556678 56 458 13666689 1334 79 13366
This coding scheme has the following rules:
1. The scheme is case-insensitive (does not distinguish between upper case and lower case letters).
2. Each letter has a unique code which is a single digit from among 1,2,3,......,9.
3. The digit 9 codes two letters, and every other digit codes three letters.
4. The code for a word is constructed by arranging the digits corresponding to its letters in a non-decreasing sequence.
Answer these questions on the basis of this information
What best can be concluded about the code for the letter L?
Video Explanation
Explanatory Answer
Digits 1 to 8 are mapped to 3 letters each, 9 is mapped to only 2.
(3 × 8) + 2 = 26 which is the total number of letters in English.
Each letter is mapped to a unique code, but remember that each number is mapped
to 2323 letters.
It is best to start from the small words. Let us attack ‘is’ and ‘as’ first.
IS = 35, AS = 56. S = 5 , I = 3 , A = 6.
Now, THE is 458. OF = 79. PEACOCK does not have a ‘7’ in in. It has an O but no F, so F should be
7 and O should be 9.
THE has 458, DESIGNATED has T and E but no H. Is there a number in THE but not in
DESIGNATED?
H should be 4. T and E should be 5 and 8 in some order.
Now let us look at INDIA. INDIA = 13366. I = 3 and A = 6. So, N and D should be 1 and 6 in some
order. BIRD has a D, BIRD = 1334.
What does this mean? This tells us D = 1 and N = 6.
Let us have another look at T and E. T and E and 5 and 8 in some order. Is there a word that has
only one of T & E but not both?
NATIONAL has T but not E. NATIONAL has 8 but not 5. BINGO! We know T has to be 8 and E has
to be 5.
Now let us go word by word. PEACOCK = P56C9CK. So, PCCK should be 8899. 9 is allotted only
to two numbers.
We know that O = 9. So, what can we say about 9?
If C takes 8, then both P and K becomes 9 which is not possible as 9 can be assigned to only two
letters.
Therefore C has to take 9 and P , K takes the value 8.
DESIGNATED = 1553G66851. The missing number should be G. Or, G = 7.
NATIONAL = 6683966L. The missing number should be L. Or, L should be 1.
BIRD = B3R1. 3 and 4 are missing. B and R should be 3 and 4 in some order.
B and R occur only once each in this sequence, so there is no way of resolving this.
From the above inferences, we can see that L takes 1.
What best can be concluded about the code for the letter B?
Video Explanation
Explanatory Answer
Digits 1 to 8 are mapped to 3 letters each, 9 is mapped to only 2.
(3 × 8) + 2 = 26 which is the total number of letters in English.
Each letter is mapped to a unique code, but remember that each number is mapped
to 2323 letters.
It is best to start from the small words. Let us attack ‘is’ and ‘as’ first.
IS = 35, AS = 56. S = 5 , I = 3 , A = 6.
Now, THE is 458. OF = 79. PEACOCK does not have a ‘7’ in in. It has an O but no F, so F should be
7 and O should be 9.
THE has 458, DESIGNATED has T and E but no H. Is there a number in THE but not in
DESIGNATED?
H should be 4. T and E should be 5 and 8 in some order.
Now let us look at INDIA. INDIA = 13366. I = 3 and A = 6. So, N and D should be 1 and 6 in some
order. BIRD has a D, BIRD = 1334.
What does this mean? This tells us D = 1 and N = 6.
Let us have another look at T and E. T and E and 5 and 8 in some order. Is there a word that has
only one of T & E but not both?
NATIONAL has T but not E. NATIONAL has 8 but not 5. BINGO! We know T has to be 8 and E has
to be 5.
Now let us go word by word. PEACOCK = P56C9CK. So, PCCK should be 8899. 9 is allotted only
to two numbers.
We know that O = 9. So, what can we say about 9?
If C takes 8, then both P and K becomes 9 which is not possible as 9 can be assigned to only two letters.
Therefore C has to take 9 and P , K takes the value 8.
DESIGNATED = 1553G66851. The missing number should be G. Or, G = 7.
NATIONAL = 6683966L. The missing number should be L. Or, L should be 1.
BIRD = B3R1. 3 and 4 are missing. B and R should be 3 and 4 in some order.
B and R occur only once each in this sequence, so there is no way of resolving this.
From the above inferences, we can see that B takes either 3 or 4.
For how many digits can the complete list of letters associated with that digit be identified?
Video Explanation
Explanatory Answer
Digits 1 to 8 are mapped to 3 letters each, 9 is mapped to only 2.
(3 × 8) + 2 = 26 which is the total number of letters in English.
Each letter is mapped to a unique code, but remember that each number is mapped
to 2323 letters.
It is best to start from the small words. Let us attack ‘is’ and ‘as’ first.
IS = 35, AS = 56. S = 5 , I = 3 , A = 6.
Now, THE is 458. OF = 79. PEACOCK does not have a ‘7’ in in. It has an O but no F, so F should be
7 and O should be 9.
THE has 458, DESIGNATED has T and E but no H. Is there a number in THE but not in
DESIGNATED?
H should be 4. T and E should be 5 and 8 in some order.
Now let us look at INDIA. INDIA = 13366. I = 3 and A = 6. So, N and D should be 1 and 6 in some
order. BIRD has a D, BIRD = 1334.
What does this mean? This tells us D = 1 and N = 6.
Let us have another look at T and E. T and E and 5 and 8 in some order. Is there a word that has
only one of T & E but not both?
NATIONAL has T but not E. NATIONAL has 8 but not 5. BINGO! We know T has to be 8 and E has
to be 5.
Now let us go word by word. PEACOCK = P56C9CK. So, PCCK should be 8899. 9 is allotted only
to two numbers.
We know that O = 9. So, what can we say about 9?
If C takes 8, then both P and K becomes 9 which is not possible as 9 can be assigned to only two
letters.
Therefore C has to take 9 and P , K takes the value 8.
DESIGNATED = 1553G66851. The missing number should be G. Or, G = 7.
NATIONAL = 6683966L. The missing number should be L. Or, L should be 1.
BIRD = B3R1. 3 and 4 are missing. B and R should be 3 and 4 in some order.
B and R occur only once each in this sequence, so there is no way of resolving this.
From
the above inferences, we can see that only two letters can be associated with digits for sure.
Which set of letters CANNOT be coded with the same digit?
Video Explanation
Explanatory Answer
Digits 1 to 8 are mapped to 3 letters each, 9 is mapped to only 2.
(3 × 8) + 2 = 26 which is the total number of letters in English.
Each letter is mapped to a unique code, but remember that each number is mapped
to 2323 letters.
It is best to start from the small words. Let us attack ‘is’ and ‘as’ first.
IS = 35, AS = 56. S = 5 , I = 3 , A = 6.
Now, THE is 458. OF = 79. PEACOCK does not have a ‘7’ in in. It has an O but no F, so F should be
7 and O should be 9.
THE has 458, DESIGNATED has T and E but no H. Is there a number in THE but not in
DESIGNATED?
H should be 4. T and E should be 5 and 8 in some order.
Now let us look at INDIA. INDIA = 13366. I = 3 and A = 6. So, N and D should be 1 and 6 in some
order. BIRD has a D, BIRD = 1334.
What does this mean? This tells us D = 1 and N = 6.
Let us have another look at T and E. T and E and 5 and 8 in some order. Is there a word that has
only one of T & E but not both?
NATIONAL has T but not E. NATIONAL has 8 but not 5. BINGO! We know T has to be 8 and E has
to be 5.
Now let us go word by word. PEACOCK = P56C9CK. So, PCCK should be 8899. 9 is allotted only
to two numbers.
We know that O = 9. So, what can we say about 9?
If C takes 8, then both P and K becomes 9 which is not possible as 9 can be assigned to only two
letters.
Therefore C has to take 9 and P , K takes the value 8.
DESIGNATED = 1553G66851. The missing number should be G. Or, G = 7.
NATIONAL = 6683966L. The missing number should be L. Or, L should be 1.
BIRD = B3R1. 3 and 4 are missing. B and R should be 3 and 4 in some order.
B and R occur only once each in this sequence, so there is no way of resolving this.
Consider option A S , U, V. We know that S takes 5. If both U and V takes 5 then there will be 4
letters coded to 5.
This is not possible.
Currency Exchange
The base exchange rate of a currency X with respect to a currency Y is the number of units of currency Y which is equivalent in value to one unit of currency X. Currency exchange outlets buy currency at buying exchange rates that are lower than base exchange rates, and sell currency at selling exchange rates that are higher than base exchange rates.
A currency exchange outlet uses the local currency L to buy and sell three international currencies A, B, and C, but does not exchange one international currency directly with another. The base exchange rates of A, B and C with respect to L are in the ratio 100:120:1. The buying exchange rates of each of A, B, and C with respect to L are 5% below the corresponding base exchange rates, and their selling exchange rates are 10% above their corresponding base exchange rates.
The following facts are known about the outlet on a particular day:
1. The amount of L used by the outlet to buy C equals the amount of L it received by selling C.
2. The amounts of L used by the outlet to buy A and B are in the ratio 5:3.
3. The amounts of L the outlet received from the sales of A and B are in the ratio 5:9.
4. The outlet received 88000 units of L by selling A during the day.
5. The outlet started the day with some amount of L, 2500 units of A, 4800 units of B, and 48000 units of C.
6. The outlet ended the day with some amount of L, 3300 units of A, 4800 units of B,and 51000 units of C.
How many units of currency A did the outlet buy on that day? [TITA]
Video Explanation
How many units of currency C did the outlet sell on that day?
Video Explanation
What was the base exchange rate of currency B with respect to currency L on that day? [TITA]
Video Explanation
What was the buying exchange rate of currency C with respect to currency L on that day?
Video Explanation
Grove snails as a whole are distributed all over Europe, but a specific variety of the snail, with a distinctive white-lipped shell, is found exclusively in Ireland and in the Pyrenees mountains that lie on the border between France and Spain. The researchers sampled a total of 423 snail specimens from 36 sites distributed across Europe, with an emphasis on gathering large numbers of the white-lipped variety. When they sequenced genes from the mitochondrial DNA of each of these snails and used algorithms to analyze the genetic diversity between them, they found that a distinct lineage (the snails with the white-lipped shells) was indeed endemic to the two very specific and distant places in question.
Explaining this is tricky. Previously, some had speculated that the strange distributions of creatures such as the white-lipped grove snails could be explained by convergent evolution—in which two populations evolve the same trait by coincidence—but the underlying genetic similarities between the two groups rules that out. Alternately, some scientists had suggested that the white-lipped variety had simply spread over the whole continent, then been wiped out everywhere besides Ireland and the Pyrenees, but the researchers say their sampling and subsequent DNA analysis eliminate that possibility too.
“If the snails naturally colonized Ireland, you would expect to find some of the same genetic type in other areas of Europe, especially Britain. We just don’t find them,” Davidson, the lead author, said in a press statement.
Moreover, if they’d gradually spread across the continent, there would be some genetic variation within the white-lipped type, because evolution would introduce variety over the thousands of years it would have taken them to spread from the Pyrenees to Ireland. That variation doesn’t exist, at least in the genes sampled. This means that rather than the organism gradually expanding its range, large populations instead were somehow moved en mass to the other location within the space of a few dozen generations, ensuring a lack of genetic variety.
“There is a very clear pattern, which is difficult to explain except by involving humans,” Davidson said. Humans, after all, colonized Ireland roughly 9,000 years ago, and the oldest fossil evidence of grove snails in Ireland dates to roughly the same era. Additionally, there is archaeological evidence of early sea trade between the ancient peoples of Spain and Ireland via the Atlantic and even evidence that humans routinely ate these types of snails before the advent of agriculture, as their burnt shells have been found in Stone Age trash heaps.
The simplest explanation, then? Boats. These snails may have inadvertently traveled on the floor of the small, coast-hugging skiffs these early humans used for travel, or they may have been intentionally carried to Ireland by the seafarers as a food source. “The highways of the past were rivers and the ocean–as the river that flanks the Pyrenees was an ancient trade route to the Atlantic, what we’re actually seeing might be the long lasting legacy of snails that hitched a ride as humans travelled from the South of France to Ireland 8,000 years ago,” Davidson said.
All of the following evidence supports the passage’s explanation of sea travel/trade EXCEPT:
Video Explanation
Explanatory Answer
The passage outlines several hypotheses and evidence related to white-lipped grove snails to arrive at the most convincing explanation for:
Video Explanation
Explanatory Answer
Which one of the following makes the author eliminate convergent evolution as a probable explanation for why white-lipped grove snails are found in Ireland and the Pyrenees?
Video Explanation
Explanatory Answer
In paragraph 4, the evidence that “humans routinely ate these types of snails before the advent of agriculture” can be used to conclude that:
Video Explanation
Explanatory Answer
The complexity of modern problems often precludes any one person from fully understanding them. Factors contributing to rising obesity levels, for example, include transportation systems and infrastructure, media, convenience foods, changing social norms, human biology and psychological factors. The multidimensional or layered character of complex problems also undermines the principle of meritocracy: the idea that the ‘best person’ should be hired. There is no best person. When putting together an oncological research team, a biotech company such as Gilead or Genentech would not construct a multiple-choice test and hire the top scorers, or hire people whose resumes score highest according to some performance criteria. Instead, they would seek diversity. They would build a team of people who bring diverse knowledge bases, tools and analytic skills.
Believers in a meritocracy might grant that teams ought to be diverse but then argue that meritocratic principles should apply within each category. Thus the team should consist of the ‘best’ mathematicians, the ‘best’ oncologists, and the ‘best’ biostatisticians from within the pool. That position suffers from a similar flaw.
Even with a knowledge domain, no test or criteria applied to individuals will produce the best team. Each of these domains possesses such depth and breadth, that no test can exist. Consider the field of neuroscience. Upwards of 50,000 papers were published last year covering various techniques, domains of enquiry and levels of analysis, ranging from molecules and synapses up through networks of neurons. Given that complexity, any attempt to rank a collection of neuroscientists from best to worst, as if they were competitors in the 50-metre butterfly, must fail. What could be true is that given a specific task and the composition of a particular team, one scientist would be more likely to contribute than another. Optimal hiring depends on context. Optimal teams will be diverse.
Evidence for this claim can be seen in the way that papers and patents that combine diverse ideas tend to rank as high-impact. It can also be found in the structure of the so-called random decision forest, a state-of-the-art machine-learning algorithm.
Random forests consist of ensembles of decision trees. If classifying pictures, each tree makes a vote: is that a picture of a fox or a dog? A weighted majority rules. Random forests can serve many ends. They can identify bank fraud and diseases, recommend ceiling fans and predict online dating behaviour. When building a forest, you do not select the best trees as they tend to make similar classifications. You want diversity. Programmers achieve that diversity by training each tree on different data, a technique known as bagging. They also boost the forest ‘cognitively’ by training trees on the hardest cases – those that the current forest gets wrong. This ensures even more diversity and accurate forests."
Yet the fallacy of meritocracy persists. Corporations, non-profits, governments, universities and even preschools test, score and hire the ‘best’. This all but guarantees not creating the best team. Ranking people by common criteria produces homogeneity. That’s not likely to lead to breakthroughs.
The author critiques meritocracy for all the following reasons EXCEPT that:
Video Explanation
Explanatory Answer
Which of the following conditions would weaken the efficacy of a random decision forest?
Video Explanation
Explanatory Answer
Which of the following conditions, if true, would invalidate the passage’s main argument?
Video Explanation
Explanatory Answer
On the basis of the passage, which of the following teams is likely to be most effective in solving the problem of rising obesity levels?
Video Explanation
Explanatory Answer
Which of the following best describes the purpose of the example of neuroscience?
Video Explanation
Explanatory Answer
