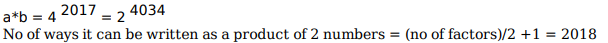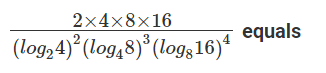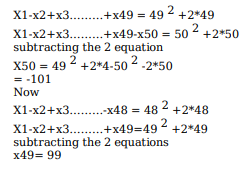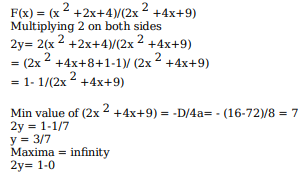Live Updates
• CATKing has launched new chat bot.

• New video on Logs has been released.
495
Learners
asked the doubt

Previous Year Questions
If x and y are non-negative integers such that x + 9 = z, y + 1 = z and x + y < z + 5, then the maximum possible value of 2x + y equals
Video Explanation

Explanatory Answer
Acc. to the question:
x + 9 = z & y + 1 = z
Therefore, x + 9 = y + 1
y = x + 8
x + y < z + 5
x + x + 8 < x + 9 + 5
x < 6
Now, 2x + y = 2x + x + 8 = 3x + 8
For this to be maximum, x has to be maximum.
Maximum possible value of x = 5.
Therefore, max possible value of 3x + 8 = 3(5) + 8 = 23
Anil buys 12 toys and labels each with the same selling price. He sells 8 toys initially at 20% discount on the labeled price. Then he sells the remaining 4 toys at an additional 25% discount on the discounted price. Thus, he gets a total of Rs 2112, and makes a 10% profit. With no discounts, his percentage of profit would have been
Video Explanation

Explanatory Answer
Let common SP be x
For 8 toys, SP = 0.8x
For remaining 4 toys, SP = 0.75*0.8x = 0.6x
Total SP = 8* 0.8x + 4* 0.6x = 2112
6.4x + 2.4x = 2112
8.8x = 2112
x = 240
Also let Total Cost Price = y
2112 = 1.1y
y = 1920
With no discounts, Total SP = 12*240 = 2880
Profit = 2880-1920 = 960
Profit % = (960/ 1920)* 100 = 50%
Let C be a circle of radius 5 meters having center at O. Let PQ be a chord of C that passes through points A and B where A is located 4 meters north of O and B is located 3 meters east of O. Then, the length of PQ, in meters, is nearest to
Video Explanation

Explanatory Answer
Since AOB is a right-angled triangle and AO = 4m, BO = 3m
AB = 5m
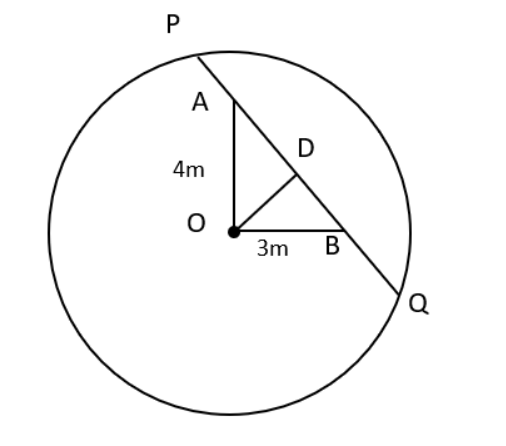
Dropping a perpendicular from O on AB at D, we know that:
OA * OB = AB * OD
4*3 = 5*OD
OD = 12/5
We know that OD bisects the chord PQ. Hence, PD = QD
Also, in triangle OPD:
OD 2 + PD 2 = OP 2
(12/5) 2 + PD 2 = 5 2 = 25
PD 2 = 25 – 144/25 = (625 – 144)/25 = 481/25
PD = Ö 481/5 ̴ 21.9/5 = 4.4
PQ = 2*PD = 8.8
For the same principal amount, the compound interest for two years at 5% per annum exceeds the simple interest for three years at 3% per annum by Rs 1125. Then the principal amount in rupees is
Video Explanation

Explanatory Answer
Let Principal Amount = x
Compound Interest = \(x(1+0.05)^{2}-x\)
= \(x(1.05)^{2}-x\)
= \(1.1025x-x\)
= \(0.1025x\)
Simple Interest = \({x*3*3 \over {100}}\)
As stated in the question,
CI = SI + 1125
0.1025x = (9x/100) + 1125
1125 = 41x/400 – 9x/100 = 5x/400 = x/80
X = 90000
![]()
Video Explanation

Explanatory Answer
log a (a/b) + log b (b/a)
= log a a - log a b + log b b - log b a
= 1 - log a b + 1 - log b a
= 2 – ( log a b + log b a )
= 2 – ( logb / loga + loga / logb )
= 2 – (x+1/x) [ loga / logb = x]
Minimum possible value of (x+1/x) is 2 , given x is + ve and real.
Hence Maximum possible value of 2 – (x+1/x) = 0 and cannot be equal to 1
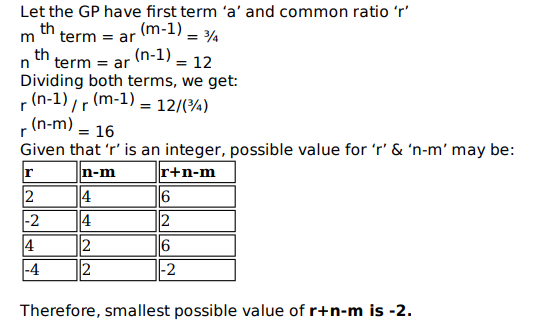
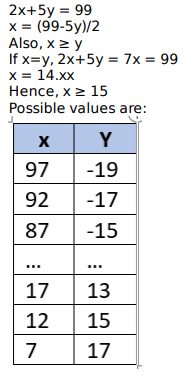
The number of pairs of integers(x,y) satisfying x ≥ y ≥ -20 and 2x + 5y = 99 is
Video Explanation

Explanatory Answer

In a group of 10 students, the mean of the lowest 9 scores is 42 while the mean of the highest 9 scores is 47. For the entire group of 10 students, the maximum possible mean exceeds the minimum possible mean by
Video Explanation

Explanatory Answer
Let a1, a2, a3, … , a10 be the 10 scores in ascending order (a1 – lowest, a10 – highest)
Mean of lowest 9 scores = 42
Sum of lowest 9 scores = 42*9 = 378 = a1+a2+ ….+ a9
Mean of highest 9 scores = 47
Sum of highest 9 scores = 47*9 = 423 = a2+a3+…+a10
Difference between highest and lowest score = 423-378 = 45 = a10-a1
Maximum possible mean when a1 is maximum
Hence a1=42, a10 = 87
Maximum mean = (378+87)/10 = 46.5
Minimum possible mean when a10 is minimum
Hence, a10=47, a1=2
Minimum mean = (423+2)/10 = 42.5
Difference = 46.5-42.5 = 4
From the interior point of an equilateral triangle, perpendiculars are drawn on all three sides. The sum of the lengths of the perpendiculars is 's'. Then the area of the triangle is
Video Explanation

Explanatory Answer
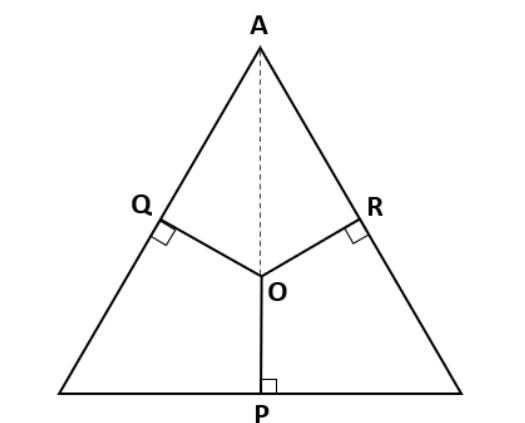
Let OP, OQ, and OR be 3 altitudes drawn from the centroid of the
equilateral triangle.
Length of all 3 p erpendicular s will be equal . Therefore, length of
OP = OQ = OR = s/3
AP is the altitude of the triangle. We know that the centroid divides
the altitude in the ratio 2:1
Hence, AO : OP = 2:1
AO = 2s/3
AP = AO + OP = s
Let side of triangle be ‘a’
Altitude AP = s = Ö 3a/2
a = 2s/ Ö 3
Area of triangle = (½)*a*s
= (½)*2s/ Ö 3*s
= s 2 / Ö 3
In a car race, car A beats car B by 45 km, car B beats car C by 50 km, and car A beats car C by 90 km. The distance (in km) over which the race has been conducted is
Video Explanation

Explanatory Answer
Answer - A
Let the length of track be ‘x’
In time ‘ t A ’, distances covered by all 3 cars are:
A: x (completes race)
B: x - 45
C: x – 90
In time ‘ t B ’, distances covered are:
B: x (Completes race)
C: x – 50
Ratio of distances = ratio of speeds
Therefore, ratio of speeds of B & C are:
\({x-45 \over {x-90}}={x \over {x-50}}\)
\((x-45)(x-50)=x(x-90)\)
\(x^{2}-95x+2250=x^{2}-90x\)
\(5x=2250\)
\(x=450\)
The points (2 , 1) and (-3 , -4) are opposite vertices of a parellelogram. If the other two vertices lie on the line x + 9y + c = 0, then c is
Video Explanation

Explanatory Answer

In a trepezium ABCD, AB is parallel to DC, BC is perpendicular to DC and ∠BAD = 45°. If DC = 5 cm, BC = 4 cm, the area of the trepezium in sq. cm is
Video Explanation

Explanatory Answer
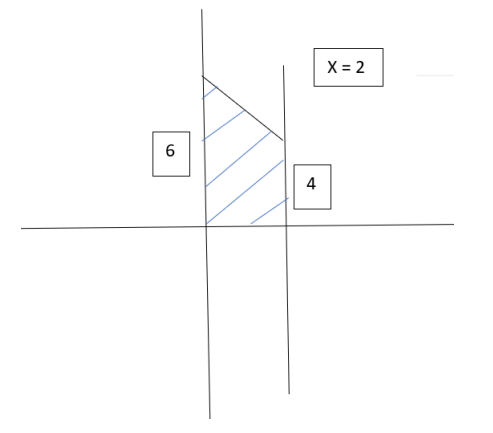
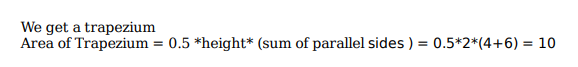
Vimla starts for office every day at 9 am and reaches exactly on time if she drives at her usual speed of 40 km/hr. She is late by 6 minutes if she drives at 35 km/hr. One day, she covers two-thirds of her distance to office in one-thirds of her usual time to reach office, and then stops for 8 minutes. The speed, in km/hr, at which she should drive the remaining distance to reach office exactly on time is
Video Explanation

Explanatory Answer

A and B are two railway stations 90 km apart. A train leaves A at 9:00 am, heading towards B at a speed of 40 km/hr. Another train leaves B at 10:30 am, heading towards A at a speed of 20 km/hr. The trains meet each other at
Video Explanation

Explanatory Answer

The vertices of a triangle are (0,0), (4,0) and (3,9). The area of the circle passing through these three points is
Video Explanation

Explanatory Answer
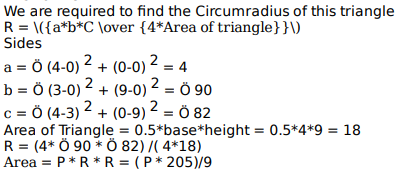
Two alcohol solutions, A and B, are mixed in the proportion 1:3 by volume. The volume of the mixture is then doubled by adding solution A such that the resulting mixture has 72% alcohol. If solution A has 60% alcohol, then the percentage of alcohol in solution B is
Video Explanation

Explanatory Answer

A batsman played n + 2 innings and got out on all occasions. His average score in these n + 2 innings was 29 runs and he scored 38 and 15 runs in the last two innings. The batsman scored less than 38 runs in each of the first n innings. In these n innings, his average score was 30 runs and lowest score was x runs. The smallest possible value of x is
Video Explanation

Explanatory Answer
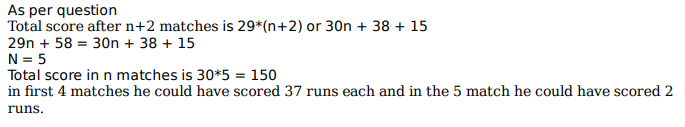
How many integers in the set {100, 101, 102, ., 999} have at least one digit repeated?
Video Explanation

Explanatory Answer

Let k be a constant. The equations kx + y = 3 and 4x + ky = 4 have a unique solution if and only if
Video Explanation

Explanatory Answer
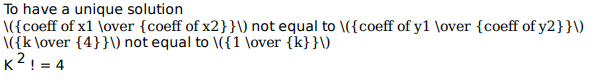
A man buys 35 kg of sugar and sets a marked price in order to make a 20% profit. He sells 5 kg at this price, and 15 kg at a 10% discount. Accidentally, 3 kg of sugar is wasted. He sells the remaining sugar by raising the marked price by p percent so as to make an overall profit of 15%. Then p is nearest to
Video Explanation

Explanatory Answer
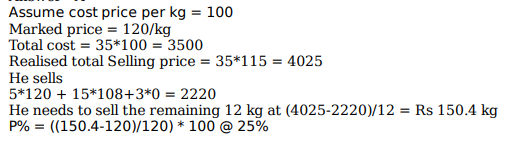
Anil, Sunil, and Ravi run along a circular path of length 3 km, starting from the same point at the same time, and going in the clockwise direction. If they run at speeds of 15 km/hr, 10 km/hr, and 8 km/hr, respectively, how much distance in km will Ravi have run when Anil and Sunil meet again for the first time at the starting point?
Video Explanation

Explanatory Answer

If f(x+y) = f(x)f(y) and f(5) = 4, then f(10) - f(-10) is equal to
Video Explanation

Explanatory Answer
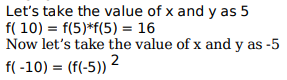
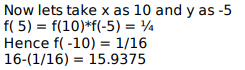
In the final examination, Bishnu scored 52% and Asha scored 64%. The marks obtained by Bishnu is 23 less, and that by Asha is 34 more than the marks obtained by Ramesh. The marks obtained by Geeta, who scored 84%, is
Video Explanation

Explanatory Answer

How many of the integers 1, 2, … , 120, are divisible by none of 2, 5 and 7?
Video Explanation

Explanatory Answer

Dick is thrice as old as Tom and Harry is twice as old as Dick. If Dick's age is 1 year less than the average age of all three, then Harry's age, in years, is
Video Explanation

Explanatory Answer
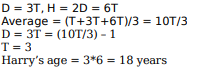
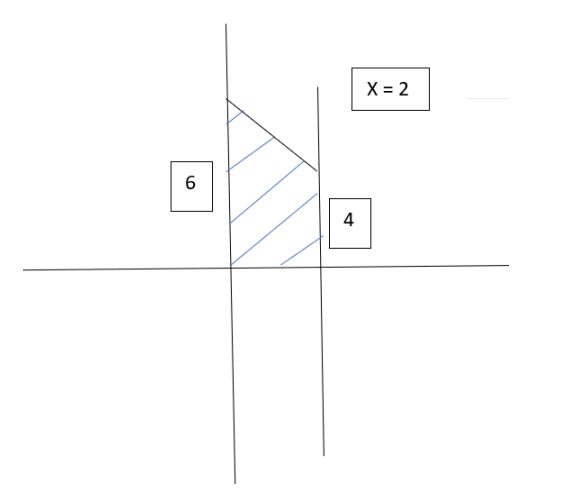
The area, in sq. units, enclosed by the lines x = 2, y = |x - 2| + 4, the X-axis and the Y-axis is equal to
Video Explanation

Explanatory Answer
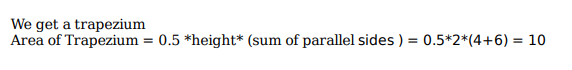
A contractor agreed to construct a 6 km road in 200 days. He employed 140 persons for the work. After 60 days, he realized that only 1.5 km road has been completed. How many additional people would he need to employ in order to finish the work exactly on time?
Video Explanation

Explanatory Answer

Let N, x and y be positive integers such that N = x + y, 2 < x < 10 and 14 < y < 23. If N > 25, then how many distinct values are possible for N?
Video Explanation

Explanatory Answer
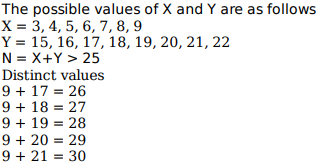

A person buys tea of three different qualities at ₹ 800, ₹ 500, and ₹ 300 per kg, respectively, and the amounts bought are in the proportion 2 : 3 : 5. She mixes all the tea and sells one-sixth of the mixture at ₹ 700 per kg. The price, in INR per kg, at which she should sell the remaining tea, to make an overall profit of 50%, is
Video Explanation

Explanatory Answer
2:3:5 and 1/6 mixture are sold
let’s assume total purchase as 60 kg
12:18:30
C.P= 9600+9000+9000= 27600
Now to get S.P. = 27600*1.5= 41400
We sold 10kg at Rs 700
41400-7000/50 = Rs 688/kg
Two pipes A and B are attached to an empty water tank. Pipe A fills the tank while pipe B drains it. If pipe A is opened at 2 pm and pipe B is opened at 3 pm, then the tank becomes full at 10 pm. Instead, if pipe A is opened at 2 pm and pipe B is opened at 4 pm, then the tank becomes full at 6 pm. If pipe B is not opened at all, then the time, in minutes, taken to fill the tank is
Video Explanation

Explanatory Answer
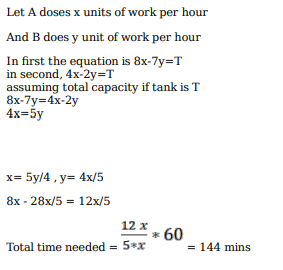
Raj invested ₹ 10000 in a fund. At the end of first year, he incurred a loss but his balance was more than ₹ 5000. This balance, when invested for another year, grew and the percentage of growth in the second year was five times the percentage of loss in the first year. If the gain of Raj from the initial investment over the two year period is 35%, then the percentage of loss in the first year is
Video Explanation

Explanatory Answer
Initial investment = Rs 10,000
Final amount = Rs 10,000*1.35 = Rs 13500
We can take an options approach to this question
Let’s assume loss percentage as 10%
The profit percentage in @nd year will be 50%
10000*0.9 = Rs 9000
9000*1.5 = Rs 13500
à 10% is the correct answer
The sides AB and CD of a trapezium ABCD are parallel, with AB being the smaller side. P is the midpoint of CD and ABPD is a parallelogram. If the difference between the areas of the parallelogram ABPD and the triangle BPC is 10 sq cm, then the area, in sq cm, of the trapezium ABCD is
Video Explanation

Explanatory Answer
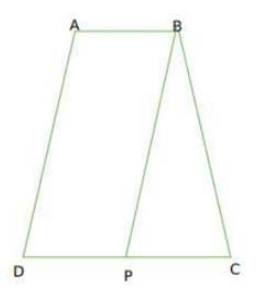
Let AB = DP = PC = x
Let the height of the parallelogram = h
Area of the parallelogram ABPD - Area of the triangle BPC = 10
xh – ½(xh) = 10
xh = 20
Area of the trapezium ABCD = xh + ½(xh) = 3/2(xh) = 30
For all real numbers x the condition |3x - 20| + |3x - 40| = 20 necessarily holds if
Video Explanation

Explanatory Answer
|3x-20| + |3x-40| = 20
If we put x=6
2+22 does not satisfy
If we put x=14
22+2 does not satisfy
à Option A, C, D can be eliminated
Hence, 7<x<12
In a football tournament, a player has played a certain number of matches and 10 more matches are to be played. If he scores a total of one goal over the next 10 matches, his overall average will be 0.15 goals per match. On the other hand, if he scores a total of two goals over the next 10 matches, his overall average will be 0.2 goals per match. The number of matches he has played is
Video Explanation

Explanatory Answer
Let no. of matches = M
Average goals = A
Total goals = MA
à (MA+1)/(M+10) = 0.15
à (MA+2)/(M+10) = 0.2
(MA+1) = 0.15(M+10) ………(i)
(MA+2) = 0.2(M+10) ………(ii)
Eq (ii)- (i)
1 = 0.05(M+10)
M = 10
For a 4-digit number, the sum of its digits in the thousands, hundreds and tens places is 14, the sum of its digits in the hundreds, tens and units places is 15, and the tens place digit is 4 more than the units place digit. Then the highest possible 4-digit number satisfying the above conditions is
Video Explanation

Explanatory Answer
Let the number be abcd
a + b + c = 14 –(1)
b + c + d = 15 –(2)
c = d + 4 – (3)
Eq (2) – (1) =
d – a = 1
d = a+1 put in eq (3)
c = a + 1 + 4
c = a + 5 –(4)
Hence, ‘a’ can take max value of 4 when c = 9
Put value of c from (4) to (1)
b + 2a + 5 = 14
b = 1 (When a is max = 4)
From (3) ‘d’ can take maximum value of 5.
Hence answer is 4195
Let D and E be points on sides AB and AC, respectively, of a triangle ABC, such that AD : BD = 2 : 1 and AE : CE = 2 : 3. If the area of the triangle ADE is 8 sq cm, then the area of the triangle ABC, in sq cm, is
Video Explanation

Explanatory Answer
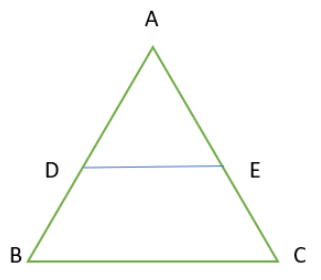
Area of Triangle ADE= (AD/AB)*(AE/AC)*(Area of ABC)
8 = (2/3)*(2/5)*(Area of ABC)
Area of ABC = 30