Live Updates
• CATKing has launched new chat bot.

• New video on Logs has been released.
495
Learners
asked the doubt

Previous Year Questions
Ramesh and Gautam are among 22 students who write an examination. Ramesh scores 82.5. The average score of the 21 students other than Gautam is 62. The average score of all the 22 students is one more than the average score of the 21 students other than Ramesh. The score of Gautam is
Video Explanation

Explanatory Answer

Consider a function f(x+y) = f(x) f(y) where x , y are positive integers, and f(1) = 2. If f (a+1) + f (a+2) + . + f(a+n) = 16 (2n - 1) then a is equal to
Video Explanation

Explanatory Answer
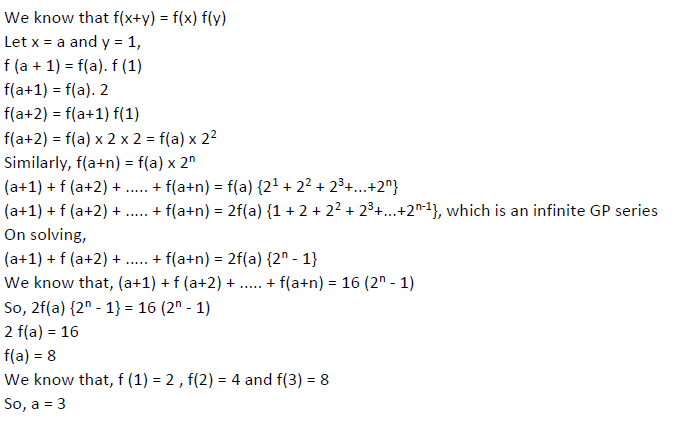
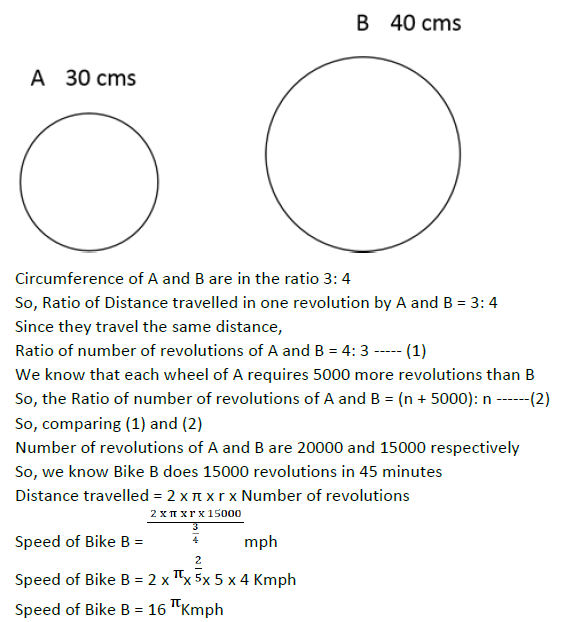
The wheels of bicycles A and B have radii 30 cm and 40 cm, respectively. While traveling a certain distance, each wheel of A required 5000 more revolutions than each wheel of B. If bicycle B traveled this distance in 45 minutes, then its speed, in km per hour, was
Video Explanation

Explanatory Answer
The product of the distinct roots of |x2 - x - 6| = x + 2 is
Video Explanation

Explanatory Answer
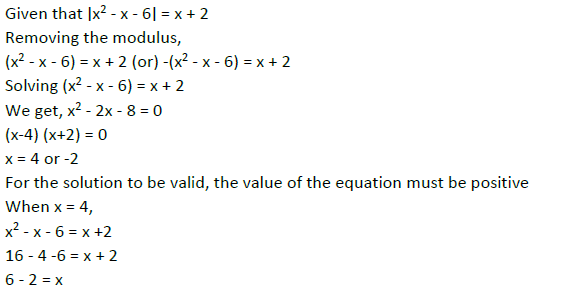

The number of solutions of the equation |x|(6x2 + 1) = 5x2 is
Video Explanation

Explanatory Answer
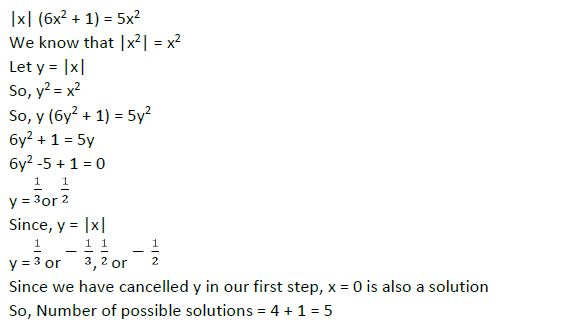
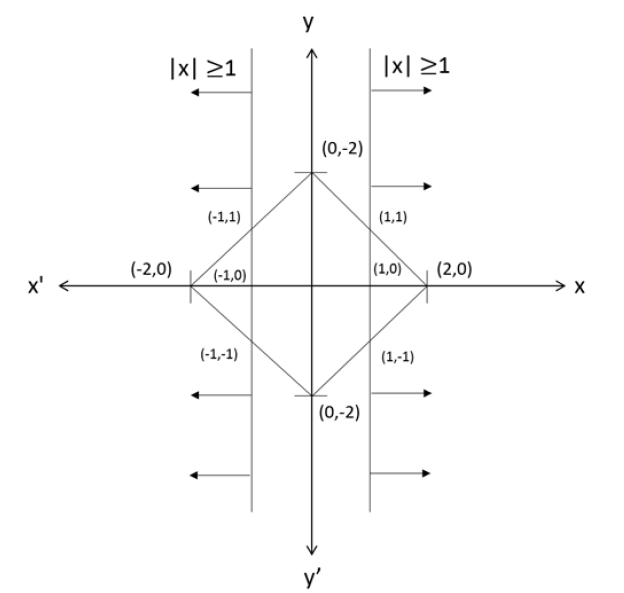
Let S be the set of all points (x,y) in the x-y plane such that |x| + |y| ≤ 2 and |x| ≥ 1. Then, the area, in square units, of the region represented by S equals
Video Explanation

Explanatory Answer

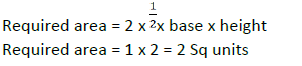
Let x and y be positive real numbers such that log5(x + y) + log5(x - y) = 3, and log2y - log2x = 1 - log23. Then xy equals
Video Explanation

Explanatory Answer
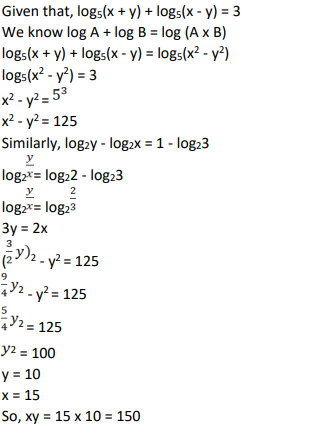
A chemist mixes two liquids 1 and 2. One litre of liquid 1 weighs 1 kg and one litre of liquid 2 weighs 800 gm. If half litre of the mixture weighs 480 gm, then the percentage of liquid 1 in the mixture, in terms of volume, is
Video Explanation

Explanatory Answer

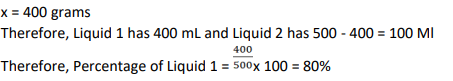
Amala, Bina, and Gouri invest money in the ratio 3 : 4 : 5 in fixed deposits having respective annual interest rates in the ratio 6 : 5 : 4. What is their total interest income (in Rs) after a year, if Bina's interest income exceeds Amala's by Rs 250?
Video Explanation

Explanatory Answer
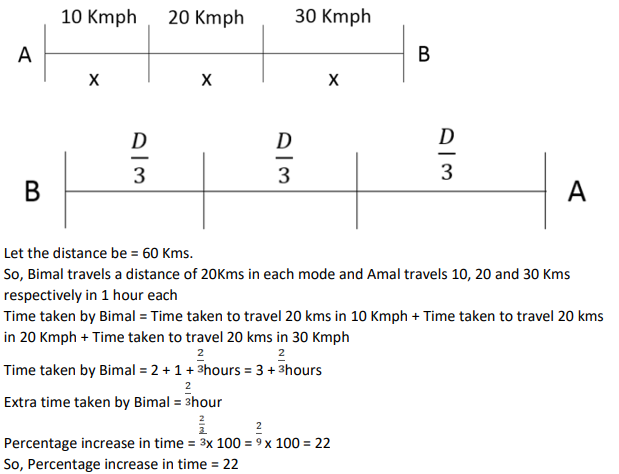
One can use three different transports which move at 10, 20, and 30 kmph, respectively. To reach from A to B, Amal took each mode of transport 1/3 of his total journey time, while Bimal took each mode of transport 1/3 of the total distance. The percentage by which Bimal's travel time exceeds Amal's travel time is nearest to
Video Explanation

Explanatory Answer
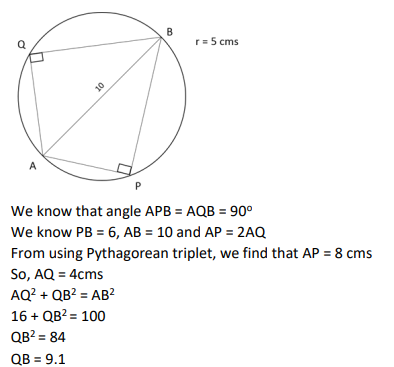
AB is a diameter of a circle of radius 5 cm. Let P and Q be two points on the circle so that the length of PB is 6 cm, and the length of AP is twice that of AQ. Then the length, in cm, of QB is nearest to
Video Explanation

Explanatory Answer
The strength of a salt solution is p% if 100 ml of the solution contains p grams of salt. If three salt solutions A, B, C are mixed in the proportion 1 : 2 : 3, then the resulting solution has strength 20%. If instead the proportion is 3 : 2 : 1, then the resulting solution has strength 30%. A fourth solution, D, is produced by mixing B and C in the ratio 2 : 7. The ratio of the strength of D to that of A is
Video Explanation

Explanatory Answer
Given that the strength of the salt solution is p% if 100 ml of the solution contains p grams of salt
It is also given that three salt solutions A , B , C are mixed in the proportion 1 : 2 : 3, then the
resulting solution has strength 20%.
So \({A+2B+3C\over6}\) = 0.2 ⟹ A + 2B + 3C = 1.2 ------(1)
If instead the proportion is 3 : 2 : 1, then the resulting solution has strength 30%.
So, \({3A+2B+C\over6}\) = 0.3 ⟹ 3A +2B + C = 1.8 -------(2)
It is given that 4 th solution D is produced by mixing B and C in the ratio 2 : 7
So D = \({2B+7C\over9}\)
We have to find the ratio of the strength of D : A
Subtracting equations 1 and 2, we get
2A – 2C = 0.6 or A – C = 0.3
Since we could not find anything from the above methods, we can eliminate the number part and
get the ratio going
A + 2B + 3C = 1.2
3A + 2B + C = 1.8
So let us multiply eqn 1 and 2 with 3 and 2,
3A + 6B + 9C = 6A + 4B + 2C
2B + 7C = 3A
It is given that D = \({2B+7C\over9}\)
\({2B+7C\over9}\) = \(3A\over9\) ⟹ 2 \({2B+7C\over9}\) = A/3
Hence D = A/3
Therefore the ratio D : A = 1 : 3
If A = {62n - 35n - 1: n = 1,2,3,.} and B = {35(n-1) : n = 1,2,3,.} then which of the following is true?
Video Explanation

Explanatory Answer

For two sets A and B, let AΔB denote the set of elements which belong to A or B but not both. If P = {1,2,3,4}, Q = {2,3,5,6,}, R = {1,3,7,8,9}, S = {2,4,9,10}, then the number of elements in (PΔQ)Δ(RΔS) is
Video Explanation

Explanatory Answer
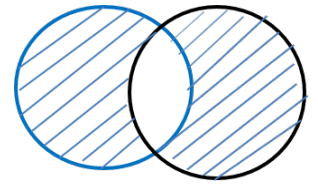
Given that for two sets A and B, A △ B denote the set of elements which belong to A or B but not
both.
This is the diagram which represents A △ B ,the set of elements which belongs to A or B i.e. A
intersection B subtracted from A union B
We have to find the number of elements in (P △ Q) △ (R △ S) if
P = {1 , 2 , 3 , 4} Q = {2 , 3 , 5 , 6 ,} R = {1 , 3 , 7 , 8 , 9} S = {2 , 4 , 9 , 10}
For P △ Q, we have to find the elements which do not to belong to both P and Q such that
(P △ Q) = {1, 4 , 5 , 6}
(R △ S) = {1 , 2 , 3 , 4 , 7 , 8 , 10}
(P △ Q) △ (R △ S) = {2 , 3 , 5 , 7 , 6 , 8 , 10}
The number of elements (P △ Q) △ (R △ S) is 7
Let f(x)=max{5x, 52 - 2x2}, where x is any positive real number.Then the minimum possible value of f(x) is
Video Explanation

Explanatory Answer


Video Explanation

Explanatory Answer
Given that AM (x, y, z) = 80
So, x + y + z = 80 × 3 = 240 ----- (1)
Also, AM (x, y, z, u, v) = 75
So, x + y + z + u + v = 75 × 5 = 375 ----- (2)
Equation (2) – (1), we get
u + v = 375 - 240 = 135
Its also given that, u = \((x+y)\over2\) and v = \((z+y)\over2\)
So, u + v = \({(x+y+z)\over2} + {y\over2}\)
\({240\over2} + {y\over2} = 135 => y = 30\)
Substituting this value in (1), we get x + z = 210
It’s given that x ≥ z
x would take the minimum value when x = z
=> 2x = 210
=> x (min) = 105
Gopal borrows Rs. X from Ankit at 8% annual interest. He then adds Rs. Y of his own money and lends Rs. X+Y to Ishan at 10% annual interest. At the end of the year, after returning Ankit’s dues, the net interest retained by Gopal is the same as that accrued to Ankit. On the other hand, had Gopal lent Rs. X+2Y to Ishan at 10%, then the net interest retained by him would have increased by Rs. 150. If all interests are compounded annually, then find the value of X + Y.
Video Explanation

Explanatory Answer
Let us draw a flow diagram to understand the transaction
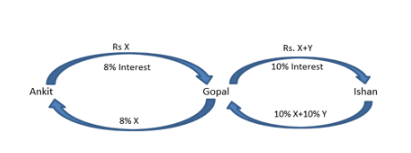
Gopal receives 10% of X + Y from Ishan as interest, from which he pays the interest of 8% of X to
Ankit
So, Gopal would gain 2% of X + 10% of Y as interest and Ankit would gain 8% Interest
Its given that both the interest values are same, so 2% X + 10% Y = 8% X
3% X = 5% Y --- (1)
Its also given that, if Gopal lend Rs. X + 2Y to Ishan at 10% interest, the net interest retained
would increase by Rs. 150
So, the increase in 10% Y accounts for Rs. 150
10% Y = 150 => Y = Rs. 1500
Substituting the value in (1), we get 5 × 1500 = 3 × X => X = 2500
So, X + Y = 2500 + 1500 = Rs 4000
A water tank has inlets of two types A and B. All inlets of type A when open, bring in water at the same rate. All inlets of type B, when open, bring in water at the same rate. The empty tank is completely filled in 30 minutes if 10 inlets of type A and 45 inlets of type B are open, and in 1 hour if 8 inlets of type A and 18 inlets of type B are open. In how many minutes will the empty tank get completely filled if 7 inlets of type A and 27 inlets of type B are open?
Video Explanation

Explanatory Answer
Let A be the rate at which pipe A fills the tank in one minute and B be the rate at which pipe B fills
in one minute
From the question, we can infer that 10A + 45B = 1/30 (Since it takes 30 minutes to fill, 1/30 th o
f the tank gets filled in 1 minute)
Similarly, we can also infer that 8A + 18B = 1/60
On simplifying, we get
2A + 9B = 1/150 ---(1)
4A + 9B = 1/120 ---(2)
Solving both, we get
2A = 1/120 - 1/150
A = 1/1200
On substituting the value of A in the equation, we get B = 1180011800
So, 7A + 27B = 7 × 1/1200 + 27 × 1/1800 = (7+18)/1200 = 1/48 th of the tank in 1 minute
So, the pipes fill the tank in 48 minutes
How many two-digit numbers, with a non-zero digit in the units place, are there which are more than thrice the number formed by interchanging the positions of its digits?
Video Explanation

Explanatory Answer
Let the two-digit number be xy, which can be expressed as 10x + y
Given that the two-digit number is more than thrice the number obtained by interchanging the
digits
So, 10x + y > 3 × (10y + x)
=> 10x + y > 30y + 3x
=> 7x > 29y
=> x > 29/7 × y
Approximately, x > 4y
Let us fix values for y and check for conditions,
For y = 1, x can take the values of 5 , 6 , 7 , 8 , 9 (As, 51 > (3 × 15), 61 > (3 ×16), 71> (3 × 17), 81
> (3 × 18), 91 > (3 × 19))
Similarly, for y = 2, the condition satisfies only for x = 9 (As, 92 > (3 × 29))
The remaining values of y does not satisfy the given conditions.
So, Total = 5 + 1 = 6 numbers
A triangle ABC has area 32 sq units and its side BC, of length 8 units, lies on the line x = 4. Then the shortest possible distance between A and the point (0,0) is
Video Explanation

Explanatory Answer
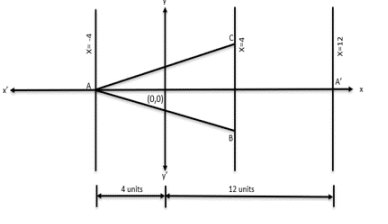
Given Area (△ABC) = 32 sq units and one of the length BC = 8 units on the line x = 4
Let us draw a graph and plot the given values.
We know that area of the Triangle = 1/2 × base × height considering BC as the base,
area of the Triangle = 1/2 × 8 × height = 32
Height = (32×2) / 8 = 64/8 = 8 units
Since the base lies on x = 4 and has a vertical height is of length = 8 units, A can either lie on the
line x = 12 or on x = - 4
However, since we need to find the shortest possible distance between A and the origin, A should
lie on the line x = - 4
So, shortest possible distance to A from the point (0,0) = 4 units
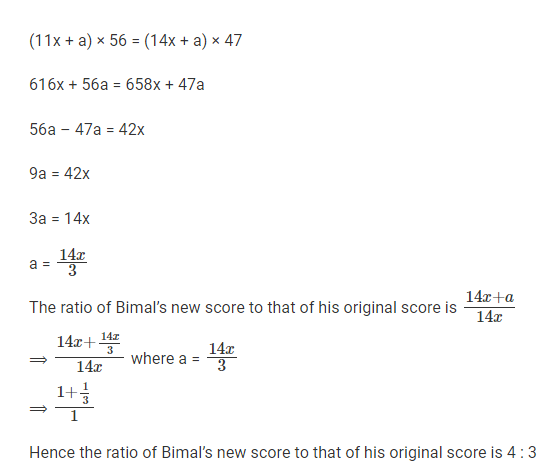
The scores of Amal and Bimal in an examination are in the ratio 11 : 14. After an appeal, their scores increase by the same amount and their new scores are in the ratio 47 : 56. The ratio of Bimal’s new score to that of his original score is
Video Explanation

Explanatory Answer


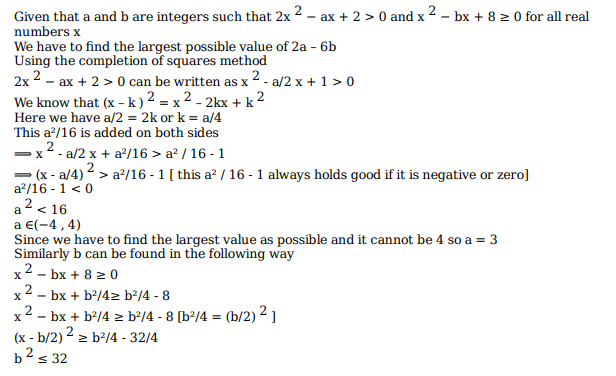
If a and b are integers such that 2x2 − ax + 2 > 0 and x2 − bx + 8 ≥ 0 for all real numbers x, then the largest possible value of 2a − 6b is
Video Explanation

Explanatory Answer

Ramesh and Ganesh can together complete a work in 16 days. After seven days of working together, Ramesh got sick and his efficiency fell by 30%. As a result, they completed the work in 17 days instead of 16 days. If Ganesh had worked alone after Ramesh got sick, in how many days would he have completed the remaining work?
Video Explanation

Explanatory Answer

Given that Ramesh and Ganesh can together complete a work in 16 days
After seven days of working together, Ramesh got sick and his efficiency fell by 30%.
To the original schedule of 16 days there are 9 more days and in this 9 days Ramesh fall short by 9
× 0.3x = 2.7x where x is the work done by Ramesh in a day
So the change = 2.7x (i.e. the gap because of drop in efficiency)
This 2.7x is compensated by one day of Ganesh + another 0.7 of Ramesh
2.7x = G + 0.7 x (Because the last day also he works with reduced efficiency)
2x = G
Hence Ganesh works twice as that of Ramesh.
If Ganesh had worked alone after Ramesh got sick means in the 7 days Ramesh would have
completed 7/48 of the task
Ganesh on each day can do 1/24 of the task
Task to be completed by Ganesh is 41/48 completely
One day he can complete 1/24 so 41/48 × 24/1 = 20.5 days
If Ganesh had worked alone after Ramesh got sick, the no.of days needed for completing the
remaining work is 20.5 – 7 = 13.5 days
A tank is emptied everyday at a fixed time point. Immediately thereafter, either pump A or pump B or both start working until the tank is full. On Monday, A alone completed filling the tank at 8 pm. On Tuesday, B alone completed filling the tank at 6 pm. On Wednesday, A alone worked till 5 pm, and then B worked alone from 5 pm to 7 pm, to fill the tank. At what time was the tank filled on Thursday if both pumps were used simultaneously all along?
Video Explanation

Explanatory Answer
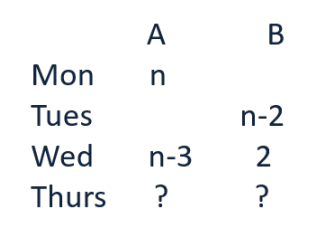
Given that a tank is emptied everyday at a fixed time point and after that either pump A or B or
both start working to fill the tank.
On Monday, A alone completed filling the tank at 8 pm.
On Tuesday, B alone completed filling the tank at 6 pm.
On Wednesday, A alone worked till 5 pm, and then B worked alone from 5 pm to 7 pm, to fill the
tank.
We have to find at what time will the tank filled on thursday if both were used simultaneously all
along
A is doing 3 hours less work on Wednesday which B completes in 2 hours therefore B = 1.5 A
⟹ (n)/(n-2) = 3/2
⟹ 2n = 3n – 6
⟹ n = 6
A takes 6 hours and B takes 4 hours or the tank is closed at 2 pm
When they are together open
⟹ 1/6 + 1/4 = (2+3)/12
⟹ Rate is 5/12 or they can fill the entire tank in 12/5 hours which is 2 hours 24 minutes
Starting from 2 pm, they fill the entire tank by 4:24 pm
Here the break through is figuring out the ratio or efficiency of the rate at which they fill is B : A
= 3 : 2
The conventional way is
⟹ (n−3)/n + 2/(n-2) = 1
n-3 hours at the rate of 1/𝑛 per hour
2 hours at the rate 1/(𝑛−2) per hour
Solving this we get n = 6 and we can find solution using this n.
Points A and B are 150 km apart. Cars 1 and 2 travel from A to B, but car 2 starts from A when car 1 is already 20 km away from A. Each car travels at a speed of 100 kmph for the first 50 km, at 50 kmph for the next 50 km, and at 25 kmph for the last 50 km. The distance, in km, between car 2 and B when car 1 reaches B is
Video Explanation

Explanatory Answer
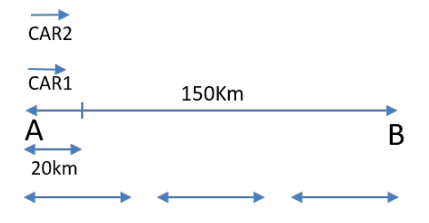
Given that points A and B are 150 km apart.
Cars 1 and 2 travel from A to B, but car 2 starts from A when car 1 is already 20 km away from A.
Each car travels at a speed of 100 kmph for the first 50 km, at 50 kmph for the next 50 km, and at
25 kmph for the last 50 km.
The Car 1 is 20km away from A and it travels at 100kmph and the time taken is 20/100 = 1/5hr
So car 2 is 12 minutes behind car 1.
We have to find the distance in km, between car 2 and B when car 1 reaches B.
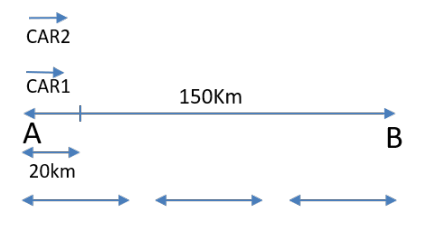
If car 1 reaches B, car 2 will take 12 minutes to reach b.
Distance between car 2 and B is 25 × 1/5 = 5 kms
The distance, in km, between car 2 and B when car 1 reaches B is 5 kms
A jar contains a mixture of 175 ml water and 700 ml alcohol. Gopal takes out 10% of the mixture and substitutes it by water of the same amount. The process is repeated once again. The percentage of water in the mixture is now
Video Explanation

Explanatory Answer
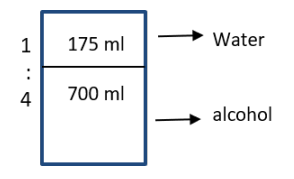
Given that a jar contains a mixture of 175 ml water and 700 ml alcohol.
It is given that 10% of the mixture is removed and it is substituted by water of the same amount
and the process is repeated once again
Now we have to find the percentage of water in the mixture.
Since the mixture is removed and substituted with water, we can deal with alcohol and the second
step we can find how much amount of alcohol is retained and not about how much amount of
alcohol is removed
As 10% of alcohol is removed, 90% of alcohol is retained
So alcohol remaining = 700 × 90% × 90%
⟹ 700 × 0.9 × 0.9 = 567
We totally have 875 ml overall mixture and of this 567 ml is alcohol.
Remaining 875 – 567 = 308 is the amount of water.
We have to find the percentage of water in the mixture i.e. 308/875
Approximately 308 is 30% of 1000 so by this we know that 308 is more than 30%
Hence 35.2% is the percentage of water in the given mixture.
If the sum of squares of two numbers is 97, then which one of the following cannot be their product?
Video Explanation

Explanatory Answer
Given that the sum of squares of two numbers is 97 i.e. a 2 + b 2 = 97
From the given options we have to find which one cannot be their product i.e. ab
A. 64 ⟹ 2ab = 128
B. −32 ⟹ 2ab = -64
C. 16 ⟹ 2ab = 32
D. 48 ⟹ 2ab = 96
2ab is found because we know that
a 2 + b 2 + 2ab ≥ 0
a 2 + b 2 - 2ab ≥ 0
By this we can know that 97 + 128 works but 97 - 128 doesn’t works so we can understand option
A cannot be the product and the rest can be.
a 2 + b 2 ≥ |2ab|
a 2 + b 2 ≥ 2ab
a 2 + b 2 ≥ -2ab
⟹( a 2 + b 2 ) / 2 ≥ |ab|
So here 2ab should lie between +97 and -97 or ab should be less than 97/2 or greater than −97/2,
so except option A all the other options works so option A 64 cannot be the product
On a long stretch of east-west road, A and B are two points such that B is 350 km west of A. One car starts from A and another from B at the same time. If they move towards each other, then they meet after 1 hour. If they both move towards east, then they meet in 7 hrs. The difference between their speeds, in km per hour, is
Video Explanation

Explanatory Answer

Given that A and B are two points such that B is 350 km west of A. One car starts from A and
another from B at the same time.
They meet after one hour or their relative velocity is (a + b)km/hr and their relative distance is
350kms
a + b = 350 which is equal to 350 kms/hr

If they both move towards east, then they meet in 7 hrs.
We have to find the difference between their speeds, in km per hour. B is catching upon A, so once
again their relative distance will be 350kms.
Relative speed = b – a , B travels faster so that it can meet a.
⟹ 350/ 𝑏−𝑎 = 7
⟹ b - a = 50 km/hr
We know that b + a = 350 km/hr
By this we can find the values of a and b but they have asked for only the difference between their
speeds which is b – a = 50km/hr
The value of the sum 7 x 11 + 11 x 15 + 15 x 19 + . + 95 x 99 is
Video Explanation

Explanatory Answer



A parallelogram ABCD has area 48 sqcm. If the length of CD is 8 cm and that of AD is s cm, then which one of the following is necessarily true?
Video Explanation

Explanatory Answer
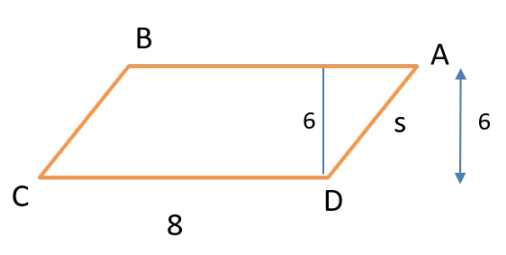
Given that a parallelogram ABCD has an area 48 sq cm. The length of CD is 8 cm and that of AD is s cm
Since the area given here is 48 sq.cm the height can be found as 6 sq.cm
We have to find which of the following options is necessarily true

Since this (shaded portion) is a right angled triangle with 6 as its height and s as its hypotenuse so we can assure that s ≥ 6 and s cannot be less than 6 so we can eliminate the other options.
By this we can come to the conclusion that s ≥ 6
The smallest integer n such that n3 - 11n2 + 32n - 28 > 0 is
Video Explanation

Explanatory Answer
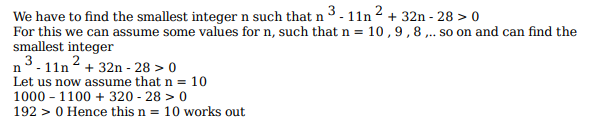
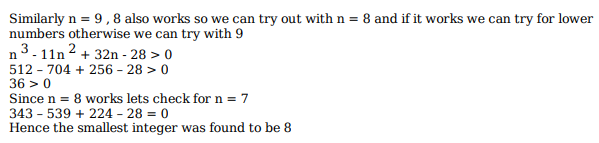

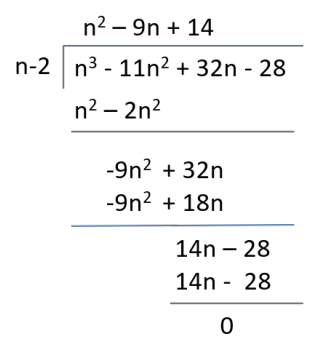
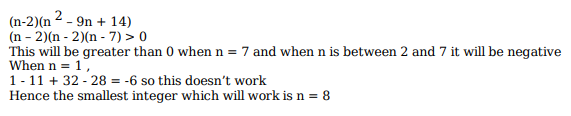
The smallest integer n for which 4n > 1719 holds, is closest to
Video Explanation

Explanatory Answer
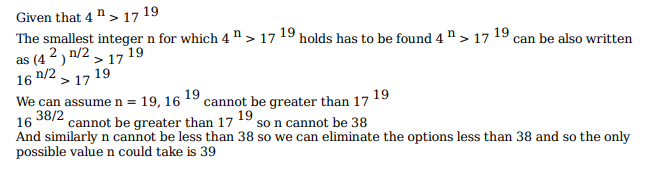
The area of a rectangle and the square of its perimeter are in the ratio 1 : 25. Then the lengths of the shorter and longer sides of the rectangle are in the ratio
Video Explanation

Explanatory Answer


A 20% ethanol solution is mixed with another ethanol solution, say, S of unknown concentration in the proportion 1:3 by volume. This mixture is then mixed with an equal volume of 20% ethanol solution. If the resultant mixture is a 31.25% ethanol solution, then the unknown concentration of S is
Video Explanation

Explanatory Answer
Given that 20% ethanol solution is mixed with another ethanol solution, say, S of unknown concentration in the proportion 1:3 by volume

This M is then mixed with an equal volume of 20% ethanol solution. If the resultant mixture is a 31.25% ethanol solution ,then the unknown concentration of S has to be found

Equal quantities of mixture and 20% ethanol solution are mixed in equal ratio to get 31.25%
The mixture and 20% ethanol solution are mixed in equal ratio to get 31.25%. This 31.25% is 11.25% more than this 20%. This mixture must be 11.25% more than 31.25% so mixture is equal to 42.5%

This mixture 42.5% is mixed in the ratio 1:3

Using allegations we can find this 22.5% which should be in the ratio 1:3 so the other one is 7.5%
S = 42.5 + 7.5
S = 50%
Here to find the solution, do the second thing first and then go to the first thing
The second one is mixed in the ratio 1 : 1 so that the final thing should be bang in the middle
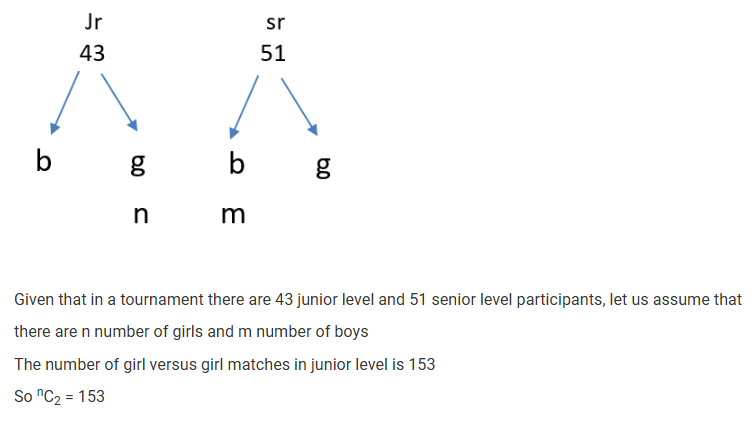
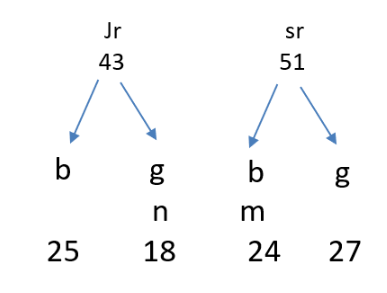
In a tournament, there are 43 junior level and 51 senior level participants. Each pair of juniors play one match. Each pair of seniors play one match. There is no junior versus senior match. The number of girl versus girl matches in junior level is 153, while the number of boy versus boy matches in senior level is 276. The number of matches a boy plays against a girl is
Video Explanation

Explanatory Answer


If p3 = q4 = r5 = s6, then the value of logs (pqr) is equal to
Video Explanation

Explanatory Answer
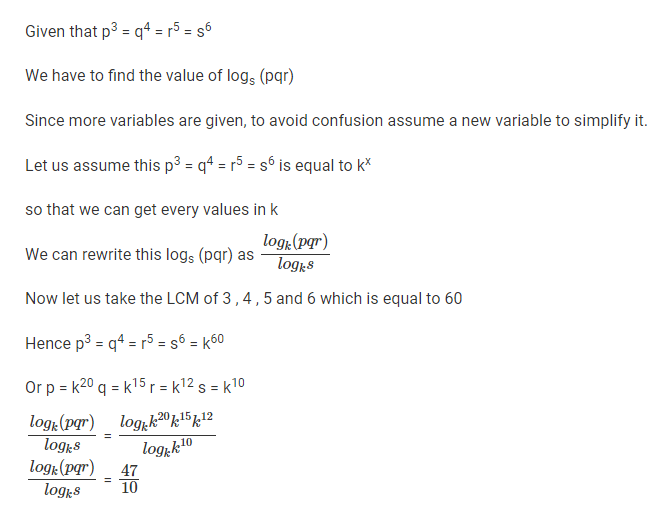
A chord of length 5 cm subtends an angle of 60° at the centre of a circle. The length, in cm, of a chord that subtends an angle of 120° at the centre of the same circle is
Video Explanation

Explanatory Answer
Given that a chord of length 5 cm subtends an angle of 60° at the centre of a circle.
We have to find the length of a chord that subtends an angle of 120° at the centre of the same
circle
sin 60° = BC/OB
⟹ √3/2 = BC/5
⟹ BC = 5√3/2 and AC = 5√3/2
So AB = 5√3/2 + 5√3/2= 5√3
The length of a chord that subtends an angle of 120° at the centre of the same circle is 5√3
If N and x are positive integers such that NN = 2160 and N2 + 2N is an integral multiple of 2x, then the largest possible x is
Video Explanation

Explanatory Answer
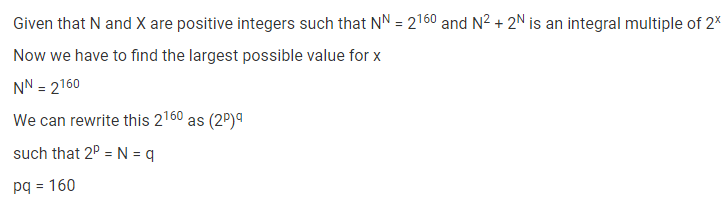


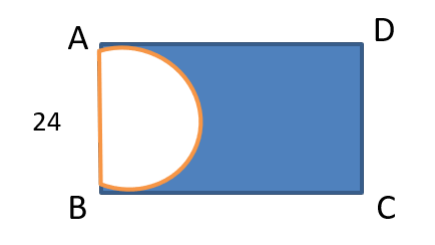
From a rectangle ABCD of area 768 sq cm, a semicircular part with diameter AB and area 72π sq cm is removed. The perimeter of the leftover portion, in cm, is
Video Explanation

Explanatory Answer

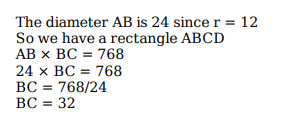

Let t1, t2,… be real numbers such that t1+ t2 +. + tn = 2n2 + 9n + 13, for every positive integer n ≥ 2. If tk=103, then k equals
Video Explanation

Explanatory Answer

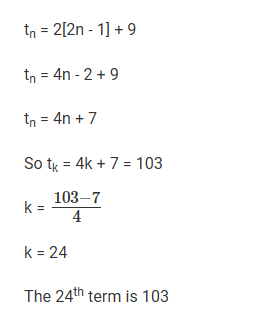
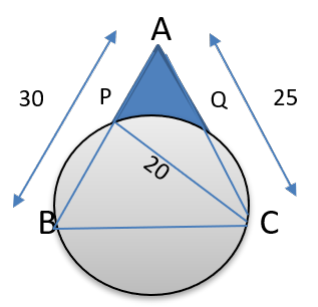
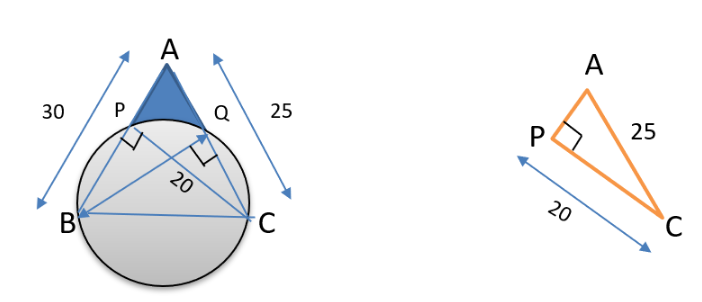
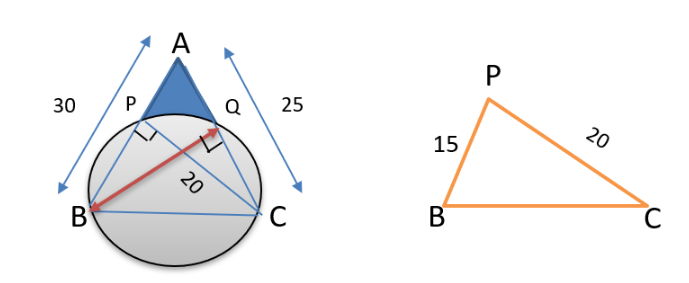
On a triangle ABC, a circle with diameter BC is drawn, intersecting AB and AC at points P and Q, respectively. If the lengths of AB, AC, and CP are 30 cm, 25 cm, and 20 cm respectively, then the length of BQ, in cm, is
Video Explanation

Explanatory Answer




There are two drums, each containing a mixture of paints A and B. In drum 1, A and B are in the ratio 18 : 7. The mixtures from drums 1 and 2 are mixed in the ratio 3 : 4 and in this final mixture, A and B are in the ratio 13 : 7. In drum 2, then A and B were in the ratio
Video Explanation

Explanatory Answer

Given that two drums each containing a mixture of paints A and B. In drum 1, A and B are in the
ratio 18 : 7. Drums 1 and 2 are mixed in the ratio 3 : 4 and in the final mixture A and B are in the
ratio 13 : 7. For these kinds of questions do not consider separately as A and B. Deal with either A
or B as a share of overall
Here A is 18/25 of overall
In D2, let us assume the proportion of A with respect to the overall mixture is x. 3 parts of D1 is
mixed with parts of D2 to give proportion of A of which is (13/20) th of the overall mixture. From
here on, it is weighted averages.
So, {(18/5)×3 + (x×4)} / 7 = 13/20
54/25 + 4𝑥 = 91/20
4𝑥 = 91/20 - 54/25
4𝑥 = (455−216)/100 = 239/100
𝑥 = 239/400
In drum 2, A is 239/400 so B should be the remaining. Therefore, A : B = 239 : 161
Let a1, a2, . , a52 be positive integers such that a1 < a2 < . < a52. Suppose, their arithmetic mean is one less than the arithmetic mean of a2, a3, ., a52. If a52 = 100, then the largest possible value of a1 is
Video Explanation

Explanatory Answer



Points A, P, Q and B lie on the same line such that P, Q and B are, respectively, 100 km, 200 km and 300 km away from A. Cars 1 and 2 leave A at the same time and move towards B. Simultaneously, car 3 leaves B and moves towards A. Car 3 meets Car 1 at Q, and Car 2 at P. If each car is moving in uniform speed then the ratio of the speed of Car 2 to that of Car 1 is
Video Explanation

Explanatory Answer

It is given that points A, P, Q and B lie on the same line such that P, Q and B are 100 km, 200 km and 300 km away from A. Let us draw the diagram first. All of them are on the same straight line and P, Q lie between A and B.
Cars 1 and Cars 2 leave A at the same time and move towards B. Simultaneously, Car 3 leaves B and moves towards A. Let us add more details to the diagram. C1 and C2 move towards B and C3 moves towards A. C3 meets C1 at Q and C3 meets C2 at P.

Let us assume the speeds of Car 1, 2, and 3 to be C1, C2 and C3 respectively. Car 1 is travelling quicker than Car 2. When Car 1 travels 200 km to reach Q, Car 3 has travelled 100 km or ratio of their speeds, C1 : C3 is 2 : 1.C1 and C2 move towards B and C3 moves towards A. C3 meets C1 at Q and C3 meets C2 at P.
Let us assume the speeds of Car 1, 2, and 3 to be C1, C2 and C3 respectively.When Car 3 travels 200 km to reach P, Car 2 has travelled 100 km or ratio of their speeds, C3 : C2 is 2 : 1. Now we have all the ratios. We know that C1 : C3 is 2 : 1 and C3 : C2 is 2 : 1. We can see that C3 is the common link

So, taking LCM of C3, we get,
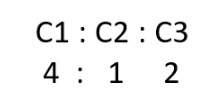
We can see that ratio of speeds of Car 2 to Car 1 is 1 : 4. Hence, C2 : C1 = 1 : 4
In an apartment complex, the number of people aged 51 years and above is 30 and there are at most 39 people whose ages are below 51 years. The average age of all the people in the apartment complex is 38 years. What is the largest possible average age, in years, of the people whose ages are below 51 years?
Video Explanation

Explanatory Answer
Given that the Average age of all the residents in the complex = 38 years
Number of people having ages 51 and above = 30
Number of people having ages below 51 ≤ 39
Since we need to maximize the average age of people below 51 and to maintain the combined average as 38 years, we need to consider the ages of people above 51 as 51 (Any more than that would lower the other value)
Also, We need to take the Number of people having ages below as 39 (Again, for maximizing the value)

So, 13 : 38-x = 39 : 30 = 13 : 10
38 - x = 10
x = 28
Average age of people whose ages are below 51 = 28 years