Live Updates
• CATKing has launched new chat bot.

• New video on Logs has been released.
495
Learners
asked the doubt

Previous Year Questions
The amount Neeta and Geeta together earn in a day equals what Sita alone earns in 6 days. The amount Sita and Neeta together earn in a day equals what Geeta alone earns in 2 days. The ratio of the daily earnings of the one who earns the most to that of the one who earns the least is
Video Explanation

Explanatory Answer
Let's consider the one-day earnings of Neeta, Geeta, Sita to be n, g, s respectively.
The amount Neeta and Geeta together earn in a day equals what Sita alone earns in 6 days
n + g = 6(s) → eq(1)
The amount Sita and Neeta together earn in a day equals what Geeta alone earns in 2 days
s + n = 2(g) → eq(2)
By solving eq (1) & (2) you will get the ratio n : g : s = 11 : 7 : 3
The ratio of the daily earnings of the one who earns the most to that of the one who earns the least is 11:3
Identical chocolate pieces are sold in boxes of two sizes, small and large. The large box is sold for twice the price of the small box. If the selling price per gram of chocolate in the large box is 12% less than that in the small box, then the percentage by which the weight of chocolate in the large box exceeds that in the small box is nearest to
Video Explanation

Explanatory Answer
Let's consider the selling prices of small and large chocolate boxes be ‘x’ and ‘2x’ respectively.
Let's also consider the weights of the small and large chocolate boxes to be 100(to make it easy) and y respectively.
Given that, the selling price per gram of chocolate in the large box is 12% less than that in the small box.
Therefore,

Hence, the weight of chocolate in a large box exceeds 127% of that of a small box.
Hence the answer is 127.
f(x) = \({x^{2}+2x-15 \over {x^{2}-7x-18}}\) is negative if and only if
Video Explanation

Explanatory Answer
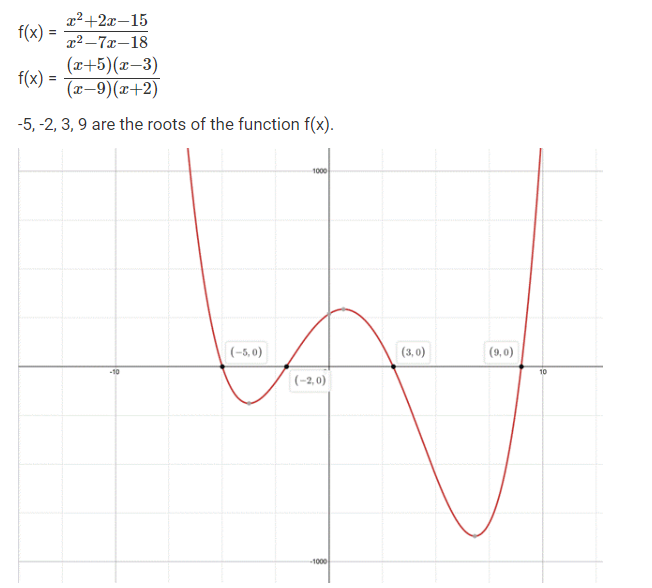
Keeping these roots on the number line and checking for the range where f(x) becomes negative.
For the numbers below -5, f(x) is positive.
For the numbers between -5 and -2, the value f(x) is negative.
For the numbers between -2 and 3, the value of f(x) is positive.
For the numbers between 3 and 9, the value of f(x) is negative.
For the numbers above 9, the value of f(x) is positive.
Hence the range should be -5 < x < -2 and 3 < x < 9.
A basket of 2 apples, 4 oranges and 6 mangoes costs the same as a basket of 1 apple, 4 oranges and 8 mangoes, or a basket of 8 oranges and 7 mangoes. Then the number of mangoes in a basket of mangoes that has the same cost as the other baskets is23301
Video Explanation

Explanatory Answer
The number of integers n that satisfy the inequalities |n-60| < |n-100| < |n-20| is
Video Explanation

Explanatory Answer
If the area of a regular hexagon is equal to the area of an equilateral triangle of side 12 cm, then the length, in cm, of each side of the hexagon is
Video Explanation

Explanatory Answer

The strength of an indigo solution in percentage is equal to the amount of indigo in grams per 100 cc of water. Two 800 cc bottles are filled with indigo solutions of strengths 33% and 17%, respectively. A part of the solution from the first bottle is thrown away and replaced by an equal volume of the solution from the second bottle. If the strength of the indigo solution in the first bottle has now changed to 21% then the volume, in cc, of the solution left in the second bottle is
Video Explanation

Explanatory Answer
Given that, there are two 800cc bottles of indigo solution with strengths of 33% and 17% respectively.
Some amount of the solution from bottle 1 is replaced by the same amount of solution from bottle 2.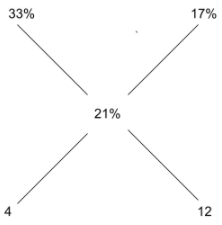
In 800cc of a new solution of strength 21% has two solutions in the ratio 1:3.
200cc of 33% solution and 600cc of 17% solution combined to give 21% solution of indigo
Hence, the remaining solution in the bottle 2 is 200cc.
Anil invests some money at a fixed rate of interest, compounded annually. If the interests accrued during the second and third year are ₹ 806.25 and ₹ 866.72, respectively, the interest accrued, in INR, during the fourth year is nearest to
Video Explanation

Explanatory Answer

Anil can paint a house in 12 days while Barun can paint it in 16 days. Anil, Barun, and Chandu undertake to paint the house for ₹ 24000 and the three of them together complete the painting in 6 days. If Chandu is paid in proportion to the work done by him, then the amount in INR received by him is
Video Explanation

Explanatory Answer

A park is shaped like a rhombus and has area 96 sq m. If 40 m of fencing is needed to enclose the park, the cost, in INR, of laying electric wires along its two diagonals, at the rate of ₹125 per m, is
Video Explanation

Explanatory Answer
The are of the rhombus is given by,
Area = ½ × d1 × d2
Where d1 & d2 are the diagonals of the rhombus.
Area = ½ × d1 × d2
96 = ½ × d1 × d2
96 × 4 = 2 × d1 × d2
Also,
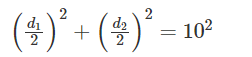
d12 + d22 = 400
(d1 + d2 )2 = d12 + d22 + 2 × d1 × d2
(d1 + d2 )2 = 400 + 4(96)
(d1 + d2 )2 = 4(100 + 96)
(d1 + d2 )2 = 4(196)
(d1 + d2) = 2(14)
d1 + d2 = 28
The cost of laying electric wires along the diagonals at the rate of ₹125 per meter
= 28 × 125
= ₹3500
The number of distinct pairs of integers (m,n) satisfying |1+mn| < |m + n| < 5 is
Video Explanation

Explanatory Answer
|1 + mn| < |m + n| < 5
For two numbers ‘a’ and ‘b’,
|a| < |b| is equivalent to a2 < b2
So, we can say that:
(1 + mn)2 < (m + n)2
1 + 2mn +m2n2 < m2 + n2 + 2mn
1 - n2 - m2 + m2n2 < 0
(1 - n2) - m2(1 - n2) < 0
(1 - m2)(1 - n2) < 0
For the product to be negative, either one of the two terms has to be negative.
But they cannot simultaneously be 0.
The only possibility for either of the two terms to be positive is when
n = 0 and |m| > 1, or |n| > 1 and m = 0
Now for the case when m = 0 and |n| > 1
|m + n| < 5
|0 + n| < 5
So n can be ±±2, ±±3, ±±4
Which are 6 cases
Similarly for the case when n = 1 and |m| > 1
|m + n| < 5
|0 + m| < 5
So m can be ±±2, ±±3, ±±4
Again we have 6 cases.
Hence the answer is 12.
Let ABCD be a parallelogram. The lengths of the side AD and the diagonal AC are 10 cm and 20 cm, respectively. If the angle ∠ADC is equal to 30° then the area of the parallelogram, in sq. cm, is
Video Explanation

Explanatory Answer
Revising the Cosine rule and the area of the triangle using the Sine rule…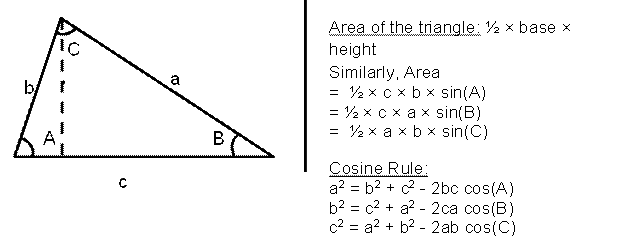
We draw the described parallelogram ABCD.

In a triangle ABC, ∠ BCA = 50°. D and E are points on AB and AC, respectively, such that AD = DE. If F is a point on BC such that BD = DF, then ∠FDE, in degrees, is equal to
Video Explanation

Explanatory Answer
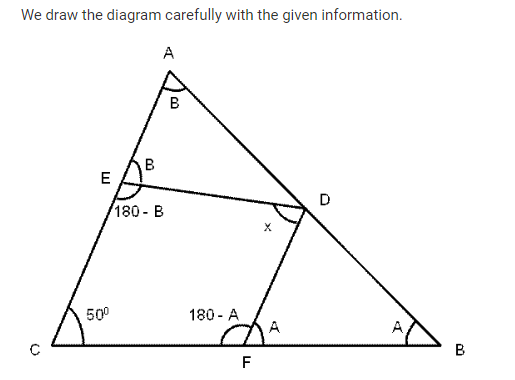
From the triangle ABC,
∠A + ∠B + ∠C = 1800
∠A + ∠B + 500 = 1800
∠A + ∠B = 1300
In the quadrilateral CFDE,
∠C + ∠F + ∠D + ∠E = 3600
500 + 1800 - ∠A + ∠x + 1800 - ∠B = 3600
500 + ∠x = ∠A + ∠B
500 + ∠x = 1300
∠x = 800
∠FDE = 800
A four-digit number is formed by using only the digits 1, 2 and 3 such that both 2 and 3 appear at least once. The number of all such four-digit numbers is
Video Explanation

Explanatory Answer
We will select the 4 digits first and arrange them later.
Out of the 4 digits, one of them should be 2 and one of them should be 3.
2, 3, , .
So, we just need to select the other two digits…
The two digits could be (1,1), (2, 2), (3, 3), (1, 2), (1, 3), or (2, 3).
So, the selection of numbers could be…
2, 3, 1, 1
2, 3, 2, 2
2, 3, 3, 3
2, 3, 1, 2
2, 3, 1, 3
2, 3, 2, 3
Each of these selections could be re-arranged in a number of ways.
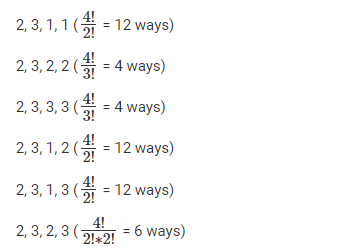
So total number of possibilities = (12 + 4 + 4 + 12 + 12 + 6) = 50 ways.
Alternate method:
(Arrangements with at least one 2 and one 3) = (All possible arrangements) - (Arrangements with either 1 or 2) - (Arrangements with either 1 or 3) + (Arrangements with only 1)
Think why we need to add (Arrangements with only 1)!
_, _, _, _
(All possible arrangements) = 34
Each blank could be any one of 1, 2 or 3.
(Arrangements with either 1 or 2) = 24
Each blank could be any one of 1 or 2.
(Arrangements with either 1 or 3) = 24
Each blank could be any one of 1 or 3.
(Arrangements with only 1) = 1
Each blank is filled with 1.
(Arrangements with at least one 2 and one 3) = (All possible arrangements) - (Arrangements with either 1 or 2) - (Arrangements with either 1 or 3) + (Arrangements with only 1)
(Arrangements with at least one 2 and one 3) = 34 - 24 - 24 + 1
(Arrangements with at least one 2 and one 3) = 81 - 16 - 16 + 1
(Arrangements with at least one 2 and one 3) = 82 - 32 = 50
A tea shop offers tea in cups of three different sizes. The product of the prices, in INR, of three different sizes is equal to 800. The prices of the smallest size and the medium size are in the ratio 2 : 5. If the shop owner decides to increase the prices of the smallest and the medium ones by INR 6 keeping the price of the largest size unchanged, the product then changes to 3200. The sum of the original prices of three different sizes, in INR, is
Video Explanation

Explanatory Answer
Since the prices of the small and medium cups are in the ratio 2 : 5,
Let us assume the prices of the small, medium and large cups to be 2x, 5x and y.
We are given that the product of the three prices is 800.
Therefore, (2x)(5x)(y) = 800 —- (1)
If the price of the smallest and the medium cups are increased by 6, then the product becomes 3200
(2x + 6) (5x + 6) (y) = 3200 —- (2)

(2x + 6) (5x + 6) = 40 x2
10 x2 + 42x + 36 = 40 x2
30 x2 - 42x - 36 = 0
10 x2 - 14x - 12 = 0
10 x2 - 20x + 6x - 12 = 0
10 x(x - 2) + 6(x - 2) = 0
(10x + 6) (x - 2) = 0
x = 2 or x = -0.6
x can’t be negative and hence x = 2
WKT, (2x)(5x)(y) = 800
(4)(10)(y) = 800
y = 20
Therefore, the sum of the prices = 2x + 5x + y
= 2(2) + 5(2) + 20
= 4 + 10 + 20
= 34
One day, Rahul started a work at 9 AM and Gautam joined him two hours later. They then worked together and completed the work at 5 PM the same day. If both had started at 9 AM and worked together, the work would have been completed 30 minutes earlier. Working alone, the time Rahul would have taken, in hours, to complete the work is
Video Explanation

Explanatory Answer
Let R be the fraction of work done by Rahul in 1 hour.
and G be the fraction of work done by Gautam in 1 hour.
Initially Rahul works from 9AM to 5PM (8 hours) and Gautam works for 2 hours less.
8R + 6G = 1 whole unit of work
If they start together they finish 30 minutes earlier or if they start at 9 AM, they finish at 4:30PM (7.5 hours)
7.5R + 7.5G = 1 whole unit of work
8R + 6G = 7.5R + 7.5G
0.5R = 1.5G
R = 3G
This means Rahul is thrice as efficient as Gautam.
8R + 6G = 1 whole unit of work
8R + 2R = 1 whole unit of work
10R = 1 whole unit of work
R is the fraction of work done by Rahul in 1 hour.
Since 10R = 1
R alone takes 10 hours to finish the job.
If a certain weight of an alloy of silver and copper is mixed with 3 kg of pure silver, the resulting alloy will have 90% silver by weight. If the same weight of the initial alloy is mixed with 2 kg of another alloy which has 90% silver by weight, the resulting alloy will have 84% silver by weight. Then, the weight of the initial alloy, in kg, is
Video Explanation

Explanatory Answer
Let the weight of Silver in the initial Alloy be Ag kgs
and the weight of Copper in the initial Alloy be Cu kgs
So, the total weight of the initial Alloy = (Ag + Cu) kgs
This Alloy is mixed with 3kgs of Pure Silver to form a new mixture.
Total weight of the mixture = (Ag + Cu) + 3 kgs
Weight of Silver in the mixture = (Ag + 3) kgs
Since this mixture contains 90% Silver,
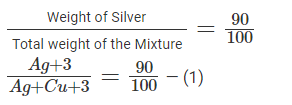
The initial Alloy is mixed with 2kgs of another alloy having 90% Silver by weight.
Total weight of the mixture = (Ag + Cu) + 2 kgs
Weight of Silver in the mixture = (Ag + 90% of 2) kgs = (Ag + 1.8) kgs
Since this mixture contains 84% Silver,
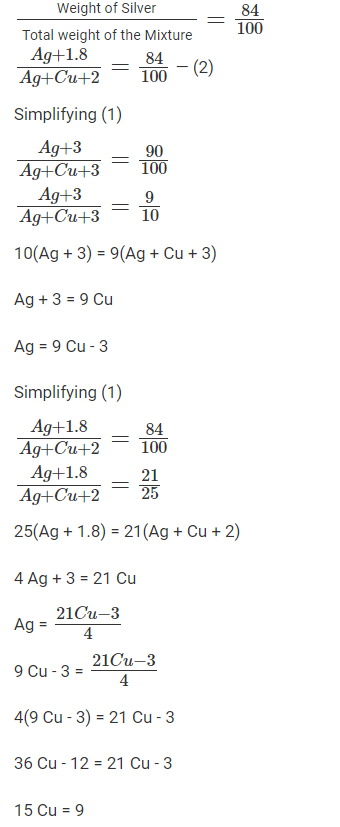
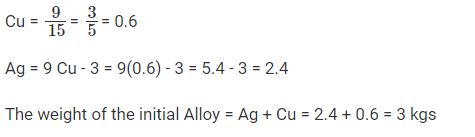
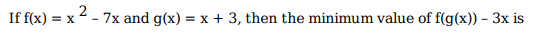
Video Explanation

Explanatory Answer
g(x) = x + 3
f(x) = x2 - 7x
f(g(x)) - 3x
= f(x + 3) - 3x
= (x + 3)2 - 7(x + 3) - 3x
= x2 + 9 + 6x - 7x - 21 - 3x
= x2 - 4x - 12
= x2 - 4x + 4 - 4 - 12
= x2 - 4x + 4 - 16
= (x - 2)2 - 16
f(g(x)) - 3x is minimum when (x - 2)2 - 16 is minimum.
(x - 2)2 - 16 is minimum when (x - 2)2 is minimum.
Since (x - 2)2 is non-negative, the minimum value it can take is 0.
Hence the minimum value of (x - 2)2 - 16 = 0 - 16 = -16
Therefore, the minimum value of f(g(x)) - 3x is -16
Bank A offers 6% interest rate per annum compounded half yearly. Bank B and Bank C offer simple interest but the annual interest rate offered by Bank C is twice that of Bank B. Raju invests a certain amount in Bank B for a certain period and Rupa invests ₹ 10,000 in Bank C for twice that period. The interest that would accrue to Raju during that period is equal to the interest that would have accrued had he invested the same amount in Bank A for one year. The interest accrued, in INR, to Rupa is
Video Explanation

Explanatory Answer
Bank A has a rate of interest of 6% and compounds half yearly.
This is the same as having a 3% interest rate per half-year.
So, if a Principal, P is invested for an year in bank A, at the end of the year it becomes P(1.03)(1.03) = P(1.0609)
Therefore the interest rate when viewed as a Simple interest scheme is 6.09% per annum.
Rupa invested in Bank C, which has twice the interest rate as Bank B and the quantum for which the investment is made is also double, hence Rupa effectively gets 4 times the interest that Raju gets for the same investment in Bank A.
Let’s say Raju invested ₹ 10,000 in Bank B.
Since this is the same as investing in Bank A for 1 year, his interest would be 6.09% of 10,000 = ₹ 609.
Now, for the same investment, Rupa must earn 4 times that of ₹ 609
So, Rupa earns ₹ 2,436
The arithmetic mean of scores of 25 students in an examination is 50. Five of these students top the examination with the same score. If the scores of the other students are distinct integers with the lowest being 30, then the maximum possible score of the toppers is
Video Explanation

Explanatory Answer
he arithmetic mean of the scores of 25 students = 50
Sum of scores of these students = 25 × 50 = 1250
For the scores of the top 5 students to be as high as possible, the score of the bottom 20 students should be as low as possible.
The minimum score is 30, and the scores of the bottom 20 students are distinct integers.
In order for the bottom 20 scores to be as low as possible, they must be,
30, 31, 32, … 49
Sum of the bottom 20 scores
= 30 + 31 + 32 + … + 49
= (30 + 0) + (30 + 1) + (30 + 2) + … + (30 + 19)
= 20 × 30 + (0 + 1 + 2 + 3 + … + 19)
= 600 + ![]()
A shop owner bought a total of 64 shirts from a wholesale market that came in two sizes, small and large. The price of a small shirt was INR 50 less than that of a large shirt. She paid a total of INR 5000 for the large shirts, and a total of INR 1800 for the small shirts. Then, the price of a large shirt and a small shirt together, in INR, is
Video Explanation

Explanatory Answer
Let the number of small shirts be ‘x’
then the number of large shirts becomes 64 - x.
Let the price of a small shirt be ‘y’
then the price of a large shirt becomes y + 50
Money spent on small shirts = xy = 1800
Money spent on large shirts = (64 - x) (y + 50) = 5000
(64 - x) (y + 50) = 5000
64y + 3200 - xy - 50x = 5000
64y + 3200 - 1800 - 50x = 5000
64y + 1400 - 50x = 5000
64y - 50x = 3600
32y - 25x = 1800
32y - 25(1800/y) = 1800
32y2 - 1800y - 25(1800) = 0
4y2 - 9(25)y - 25(9)(25) = 0
y = 75
Price of a small shirt = ‘y’ = 75
Price of a small shirt = ‘y + 50’ = 125
The price of a large shirt and a small shirt together, in INR = 75 + 125 = 200
One part of a hostel's monthly expenses is fixed, and the other part is proportional to the number of its boarders. The hostel collects ₹ 1600 per month from each boarder. When the number of boarders is 50, the profit of the hostel is ₹ 200 per boarder, and when the number of boarders is 75, the profit of the hostel is ₹ 250 per boarder. When the number of boarders is 80, the total profit of the hostel, in INR, will be
Video Explanation

Explanatory Answer
Let the fixed cost be ₹ F and the variable cost be ₹ V.
Since the profit per border is ₹200 when there are 50 borders
The expenses of the Hostel is,
F + 50(V) = 50 (1600 - 200)
F + 50(V) = 50 (1400) — (1)
Since the profit per border is ₹250 when there are 75 borders
The expenses of the Hostel is,
F + 75(V) = 75 (1600 - 250)
F + 75(V) = 75 (1350) — (2)
(2) - (1)
25(V) = 75(1350) - 50(1400)
25(V) = 25( 3(1350) - 2(1400) )
V = 3(1350) - 2(1400)
V = 4050 - 2800
V = 1250
F + 75(V) = 75 (1350)
F + 75(1250) = 75 (1350)
F = 75(100) = 7500
The Expenditure for 80 borders will be,
= F + 80(V)
= 7500 + 80(1250)
The revenue collected from 80 students is,
= 80(1600)
Hence, the profit is,
= 80(1600) - (7500 + 80(1250))
= 80(1600 - 1250) - 7500
= 80(350) - 7500
= 100(8×35 - 75)
= 20500
Hence the total profit when there are 80 borders is ₹20500.
If 3x + 2|y| + y = 7 and x + |x| + 3y = 1, then x + 2y is
Video Explanation

Explanatory Answer
3x + 2|y| + y = 7
x + |x| + 3y = 1
lets take x and y to be positive
3x+3y=7
2x+3y=1
x=6, but y is negative so this case is invalid
Lets take x is +ve and y is -ve
3x-y=7
2x+3y=1
à x=2 and y=-1
Case is valid
x+2y = 2 + 2(-1) = 0
In a tournament, a team has played 40 matches so far and won 30% of them. If they win 60% of the remaining matches, their overall win percentage will be 50%. Suppose they win 90% of the remaining matches, then the total number of matches won by the team in the tournament will be
Video Explanation

Explanatory Answer
Let the number of matches to be played be ‘x’
We are given that 40 matches are already played and 30% of them are won.
If 60% of the remaining matches are won, then the overall win percentage is 50%.
30% of 40 + 60% of x = 50% of (40 + x)
0.3 (40) + 0.6 (x) = 0.5 (40 + x)
12 + 0.6 (x) = 20 + 0.5 (x)
0.1 (x) = 8
x = 80
The number of matches to be played is 80.
If the team wins 90% of the remaining matches, it would win 90% of 80 = 72 matches.
Total matches won by the team
= 30% of 40 + 72
= 12 + 72 = 84
Mira and Amal walk along a circular track, starting from the same point at the same time. If they walk in the same direction, then in 45 minutes, Amal completes exactly 3 more rounds than Mira. If they walk in opposite directions, then they meet for the first time exactly after 3 minutes. The number of rounds Mira walks in one hour is
Video Explanation

Explanatory Answer

The total of male and female populations in a city increased by 25% from 1970 to 1980. During the same period, the male population increased by 40% while the female population increased by 20%. From 1980 to 1990, the female population increased by 25%. In 1990, if the female population is twice the male population, then the percentage increase in the total of male and female populations in the city from 1970 to 1990 is
Video Explanation

Explanatory Answer
From 1970 to 1980,
the male population increased by 40%
the female population increased by 20%
the overall population increased by 25%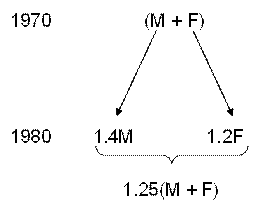
1.4M + 1.2F = 1.25(M + F)
1.4M + 1.2F = 1.25M + 1.25F
1.4M - 1.25M = 1.25F - 1.2F
0.15M = 0.05F
F = 3M
From 1980 to 1990,
Female population increased by 25%
Therefore, the female population in 1990 = 1.25 × 1.2F = 1.5F
Since, the female population in 1990 is twice the male population,
Male population in 1990 = 1.5F ÷ 2 = 0.75F
Since F = 3M,
Male population in 1990 = 2.25M
Total population in 1970 = M + F = M + 3M = 4M
Total population in 1990 = 2.25M + 1.5F = 2.25M + 4.5M = 6.75M
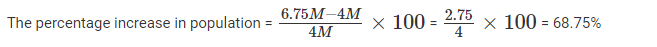
The cost of fencing a rectangular plot is ₹ 200 per ft along one side, and ₹ 100 per ft along the three other sides. If the area of the rectangular plot is 60000 sq. ft, then the lowest possible cost of fencing all four sides, in INR, is
Video Explanation

Explanatory Answer
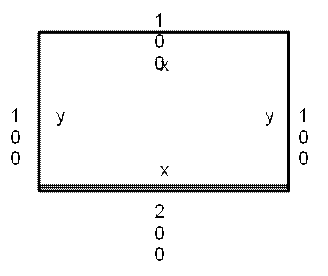
Let the length be x and the breadth be y,
Then the area of the region will be
x×y = 60000
Then the cost of fencing the region will be
200x + 100x + 100y + 100y
300x + 200y
Now we know that the Arithmetic mean ≥ Geometric mean.
The average weight of students in a class increases by 600 gm when some new students join the class. If the average weight of the new students is 3 kg more than the average weight of the original students, then the ratio of the number of original students to the number of new students is
Video Explanation

Explanatory Answer

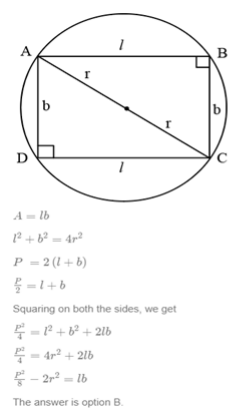
All the vertices of a rectangle lie on a circle of radius R. If the perimeter of the rectangle is P, then the area of the rectangle is
Video Explanation

Explanatory Answer
In a class of 100 students, 73 like coffee, 80 like tea and 52 like lemonade. It may be possible that some students do not like any of these three drinks. Then the difference between the maximum and minimum possible number of students who like all the three drinks is
Video Explanation

Explanatory Answer

Alex invested his savings in two parts. The simple interest earned on the first part at 15% per annum for 4 years is the same as the simple interest earned on the second part at 12% per annum for 3 years. Then, the percentage of his savings invested in the first part is
Video Explanation

Explanatory Answer

Amal buys 110 kg of syrup and 120 kg of juice, syrup being 20% less costly than juice, per kg. He sells 10 kg of syrup at 10% profit and 20 kg of juice at 20% profit. Mixing the remaining juice and syrup, Amal sells the mixture at ₹ 308.32 per kg and makes an overall profit of 64%. Then, Amal's cost price for syrup, in rupees per kg, is
Video Explanation

Explanatory Answer

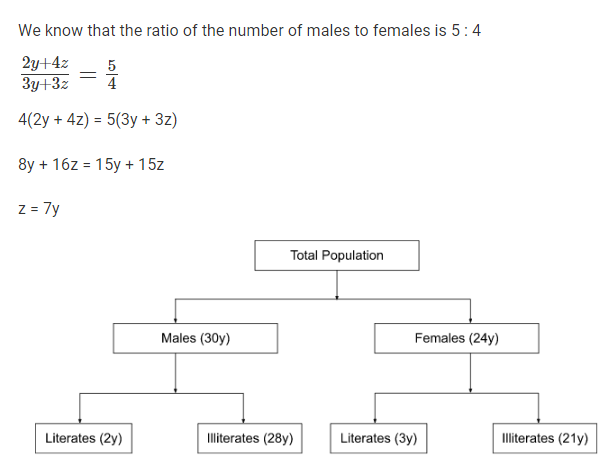
In a village, the ratio of number of males to females is 5 : 4. The ratio of number of literate males to literate females is 2 : 3. The ratio of the number of illiterate males to illiterate females is 4 : 3. If 3600 males in the village are literate, then the total number of females in the village is
Video Explanation

Explanatory Answer


A trapezium ABCD has side AD parallel to BC,∠BAD=90∘,BC=3~cm and AD=8~cm. If the perimeter of this trapezium is 36 cm, then its area, in sq. cm, is
Video Explanation

Explanatory Answer
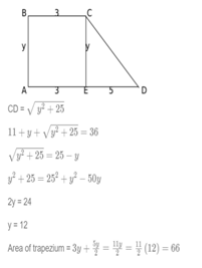
The largest real value of a for which the equation |x+a|+|x−1|=2 has an infinite number of solutions for x is
Video Explanation

Explanatory Answer

Ankita buys 4 kg cashews, 14 kg peanuts and 6 kg almonds when the cost of 7 kg cashews is the same as that of 30 kg peanuts or 9 kg almonds. She mixes all the three nuts and marks a price for the mixture in order to make a profit of ₹1752. She sells 4 kg of the mixture at this marked price and the remaining at a 20% discount on the marked price, thus making a total profit of ₹744. Then the amount, in rupees, that she had spent in buying almonds is
Video Explanation

Explanatory Answer

Pinky is standing in a queue at a ticket counter. Suppose the ratio of the number of persons standing ahead of Pinky to the number of persons standing behind her in the queue is 3 : 5. If the total number of persons in the queue is less than 300, then the maximum possible number of persons standing ahead of Pinky is
Video Explanation

Explanatory Answer

The average of three integers is 13 . When a natural number n is included, the average of these four integers remains an odd integer. The minimum possible value of n is
Video Explanation

Explanatory Answer

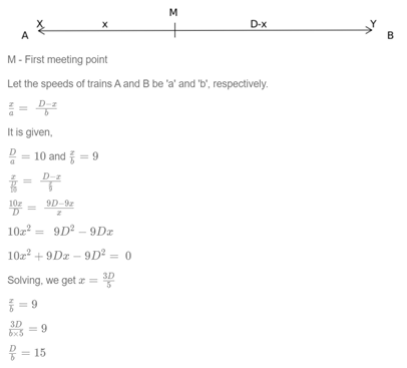
Trains A and B start traveling at the same time towards each other with constant speeds from stations X and Y, respectively. Train A reaches station Y in 10 minutes while train B takes 9 minutes to reach station X after meeting train A. Then the total time taken, in minutes, by train B to travel from station Y to station X is
Video Explanation

Explanatory Answer
A mixture contains lemon juice and sugar syrup in equal proportion. If a new mixture is created by adding this mixture and sugar syrup in the ratio 1 : 3, then the ratio of lemon juice and sugar syrup in the new mixture is
Video Explanation

Explanatory Answer